Water Hammer Calculations

[PEDARRIN2]

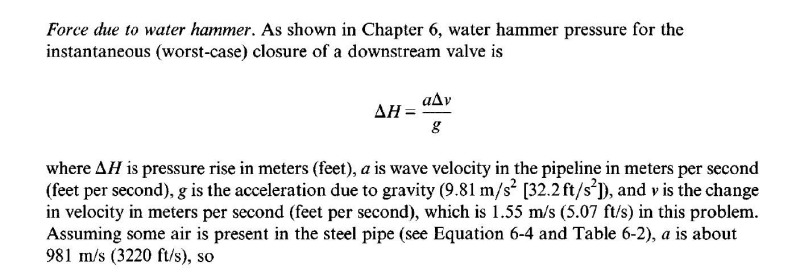
I have looked on other threads. I have looked at technical documents. I have looked at on line calculators.

I am missing something.

There seems to be two different ways to calculate the pressure and/or force exerted when flow is stopped by a quick acting valve, i.e. water hammer.

One way per thread124-18869 indicates h(wh)=a*DV/gc where a is the velocity of wave propagation, D is the inside pipe diameter, V is the change in velocity of the fluid, and gc is the grav. constant.

Then there is the other approach/method found in several locations including engineering toolbox

http://www.engineeringtoolbox.com/water-hammer-d_966.html

for water hammer which states P=0.07VL/T where V is the velocity, L is the pipe length, t is the valve closing time.

I am assuming h(wh) and 0.07VL/T are analogous in that they represent the surge in pressure on the system caused by the velocity drop, but i do not see how since they use different system variables

Assume

a = 4,000 ft/s
D = 0.5 in
V = 10 ft/s
gc = 32.2
L = 20 ft
T = 0.1 sec

Unless I am missing something, the first equation lacks the density of water (62.4 lb/cf) to make the units work and adding conversions from feet to inches so

lb/in^2 = 10*4000*0.5*62.4 / 32.2*1728

= 22.4

When I use the second equation, which the units do not get to pressure,

lb/in^2 = 0.07*10*20/0.1

= 140

What am i missing?

 

[BigInch]

The first is for an "instantaneous" valve closing time, where closing time is much faster than 2 x length / propagation velocity.

 

[LittleInch]

If you ignore the additional factors you've added, I got about 275 psig for the first one.

The L in the second equation means that the surge pressure increases at the valve when the length increases ( more mass).

Both of these are at best simple worst case scenarios and for short piping lengths probably Ok, but anything more complex or considerably longer, you need to start using a transient analysis program to model pressure wave attenuation due to the friction and the walls expanding.

This also allows the pressure wave to reflect back. It starts to get complex if your lengths and flowrates increase.

Remember - More details = better answers
Also: If you get a response it's polite to respond to it.

 

[PEDARRIN2]

Thanks for the responses.

One of my sources indicated different approaches if the valve closing was less than or greater than the t=2L/a - so I get that.

So, in general, if the valve closes quicker than 2L/a - I should probably use h(wh)=a*DV/gc , but when it is slower, I should probably use 0.07VL/T?

I am looking at trying to wrap my head around the issue of flush valves in a commercial toilet room. Typical flow each is 25 gpm but like to use 50 gpm for two "simultaneous" valves. The length of piping would be around 10-15 feet. We typically put a spring piston hammer arrestor on the piping, but some owners do not like them. We also use a larger manifold pipe to reduce the velocity, reducing the momentum of the water, but this effect is not as straight forward because increasing the pipe size will increase the volume of water which increases the momentum. I am trying to see when I should specify more pipe support to help minimize the pipe movement from the surge

I understand how to approach the problem when the valve is on a straight pipe, but with flush valves you will have a tee off the main to go into the room, then there is the elbow of the flush valve that directs the flow to the actual valve. I don't know if this would constitute use of a transient analysis program or not.

 

[LittleInch]

I always think pipelines, but once you start to look at networks you're in trouble.

My understanding has always been that if the pipe coming to a halt is connected to another pipe which is flowing, the surge wave just vanishes as soon as it hits the tee where flow is occurring. You only really get classic water hammer when you have a length of pipe which is only flowing to a single item. Elbows act to divert the shock wave along the pipe, but get a reaction force on them.

It all depends on what is flowing at the same time

Remember - More details = better answers
Also: If you get a response it's polite to respond to it.

 

[BigInch]

The wave doesn't vanish at a tee, or a reducer. Fittings act in the same manner as an orifice plate, or a partially closed valve. Anything that causes a change in hydraulic energy reflects a portion of a wave moving along a pipeline. For example a change in diameter partially reflects the wave's energy according to the ratio of upstream and downstream areas. The remaining portion of the wave passes on downstream. The Exxon Mobil Pipeline Hydraulic Manual has a good chapter devoted to those effects. In a network waves can sometimes become amplified and more complicated due to constructive or destructive wave interferences, especially those set up by steady state flows as they are more static, however transient waves can dissipate even more quickly within all the reflections.

 

[bimr]

Some rule of thumbs:

1. If the flow is less than 100 gal/min, the small diameter piping has a high pressure rating and can usually withstand the pressure.
2. Pipe network junctions significantly dissipate the pressure waves and do not need to be analyzed.
3. If the velocity is kept below 6 ft/sec, water hammer should not be a concern.
4. Pumping systems with a head greater than 50 ft. if the flow is grater than about 500 gal/min should be analyzed.

For your system, there is little that may be done to minimize water hammer except for limiting the fluid velocity.

Here is another water hammer formula from Garr Jones' Book. This formula is more intuitive.

 

[Krausen]

PEDARRIN2 - Increasing the piping/manifold size will help you here. At a constant flow rate, as your pipe size increases your flow velocity decreases. Water hammer (line pressure surge) is a function of flow velocity, not volume. As your flow velocity decreases, water hammer surge decreases.

Larger pipe size will also decrease your bulk wave speed (combined wave speed through liquid-filled pipe), which also slightly lowers potential surge pressures.

 

[sailoday28]

PEDARRIN2
Do you have piping downstream of the valve in question? If so, the t=2L/a does not consider that piping. Then you could include valve flow area vs time and downstream hydraulics.

Sailoday28

 

[PEDARRIN2]

The valve in question is a flush valve so the only piping downstream is the short piece that connects to the toilet or urinal.

The gist of what I am reading is that my pipe is not large enough, nor do I really have enough flow for water hammer to be an issue. But pipes sometimes move and they sometimes make noise, which might be called water hammer by the client or contractor, but could just be poorly supported piping in the chase behind the fixtures. Since pipe supports are generally loosely specified and not detailed, what gets installed could run the gambit.

Maybe this is more of a support issue than a flow issue.

 

[davefitz]

As I recall, there are several other factors that need to be confirmed. I think the formulas you cite assume water flowing inside steel pipes. There are other fluids, and there are other pipe materials, and their alternate use will imply that you will need to go back to first principals to compute the water hammer surge pressure.

There are also other factors to consider when determining if the surge pressure will cause damage or not; I recall that the energy of the surge must exceed the energy needed to cause damage to the pipe, so that needs to be checked.

Finally, there are simple measures to reduce the surge pressure or energy that occurs during waterhammer events , including adding a compressible bladder in the pipe system, limiting the speed of closure for the last 5% of valve stroke, and preventing full tight closure. However, programming these tricks in the control system logic is not a failsafe solution; the fatal pipeline failure in Washington state in 1999 was partly caused by a reset of the redundant control systems; during reset it led to a failed -closed fast closure of the stop valves in a 100 mile long gasoline pipe( underground), and the change in momentum of a 100 mile leg of gasoline led to the failure of the pipe at a location was previously damaged by a backhoe.

"Nobody expects the Spanish Inquisition!"