David Ren
Student
- Mar 22, 2024
- 5
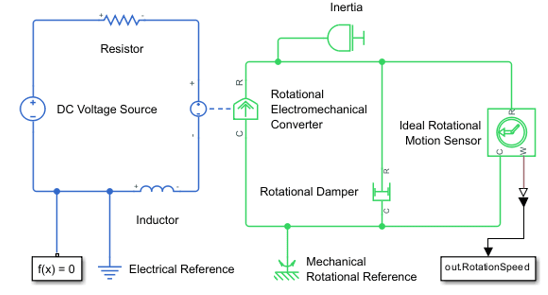
I have a Simulink model of an open-loop DC motor.
I am trying to understand what parameters effect the final steady state angular velocity of the motor.
From playing around with different values, it seems that:
A. The input voltage, electric resistance, viscous resistance (damper) and torque constant have an effect on the steady state velocity
B. The Inertia and electric inductance do not affect the final speed, but rather only effect the time it takes to reach this speed.
Am I correct? Why is this? my intuition says that also the inertia should influence the steady state speed, but it seems my intuition is wrong.
Thanks!
I am trying to understand what parameters effect the final steady state angular velocity of the motor.
From playing around with different values, it seems that:
A. The input voltage, electric resistance, viscous resistance (damper) and torque constant have an effect on the steady state velocity
B. The Inertia and electric inductance do not affect the final speed, but rather only effect the time it takes to reach this speed.
Am I correct? Why is this? my intuition says that also the inertia should influence the steady state speed, but it seems my intuition is wrong.
Thanks!

