RipleyGallegos
Mechanical
A simple pump that lifts 1,178 gallons per lever stroke. I ask this question because the bottom chamber has a larger diameter than the cylinder it's pumping water up into.(PHOTO) The math makes sence on a seesaw drawing but I'm unsure with this pump due to the different sized cylinders as friction will occur.
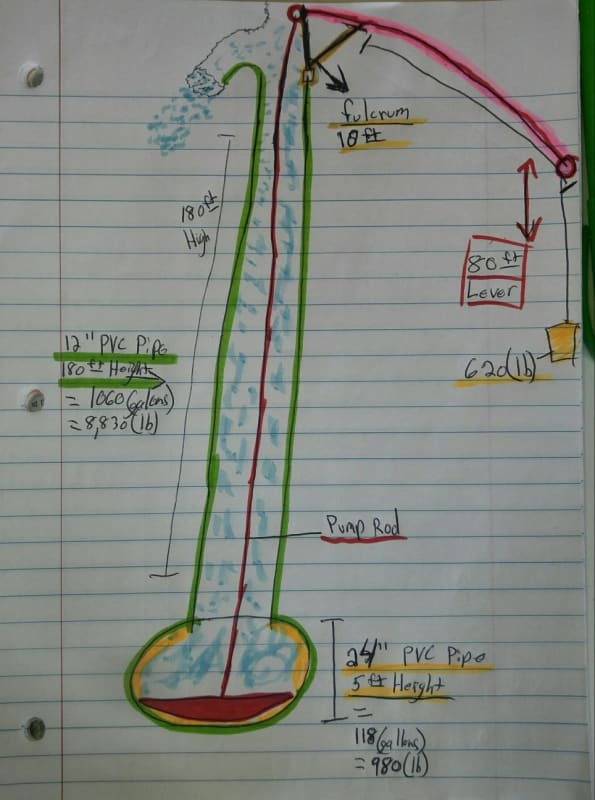
The lever is 80 ft long with 620(lb) load at the very end. The other end of the FULCRUM is 10ft long.
At this end is the pump rod & the piston in the bottom chamber needing to lift approximately 1,178 gallons (which is the amount of water in the well. = 9,810(lb). Here's the math with a drawn photo of the pump.
Bottom chamber = 24inch PIPE 5ft High. = 15.7 ft(3) or 118 gallons. =980(lb)
Smaller chamber is half the diameter. = 12 inch PIPE 180ft High = 141ft(3) or 1,060 gallons. =8,830(lb)
Together a total of = 9,810 (lb) must be lifted.
Lever is 10ft by (FULCRUM) 80ft long. With a 620(lb) load at the very end.
Again on,a seesaw this would work but will this pump work even though there will be friction from the different cylinder sizes? Thanks!
The lever is 80 ft long with 620(lb) load at the very end. The other end of the FULCRUM is 10ft long.
At this end is the pump rod & the piston in the bottom chamber needing to lift approximately 1,178 gallons (which is the amount of water in the well. = 9,810(lb). Here's the math with a drawn photo of the pump.
Bottom chamber = 24inch PIPE 5ft High. = 15.7 ft(3) or 118 gallons. =980(lb)
Smaller chamber is half the diameter. = 12 inch PIPE 180ft High = 141ft(3) or 1,060 gallons. =8,830(lb)
Together a total of = 9,810 (lb) must be lifted.
Lever is 10ft by (FULCRUM) 80ft long. With a 620(lb) load at the very end.
Again on,a seesaw this would work but will this pump work even though there will be friction from the different cylinder sizes? Thanks!