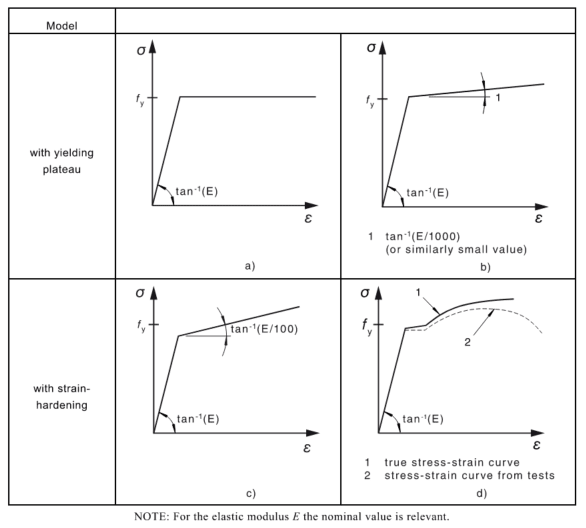
I am trying to learn more about FEA using nonlinear materials to assess the capacity of steel structures, components, brackets, etc.
I understand that when using linear-elastic analysis around sharp notches, re-entrant corners, and the like, there will always be a stress concentration that approaches infinite stress with increasing mesh refinement. I realise also that in a real structure, the geometry never really comes to a perfectly sharp corner if we 'zoom in' far enough, and in any case, the material will locally yield around those stress concentrations and tend to 'even out' the large stress peak predicted by the linear-elastic analysis.
I suppose my first question is this: if we use a nonlinear material in the FEA (e.g., assume an elastic-perfectly plastic stress-strain curve for steel), how do we then interpret the stresses around notches, re-entrant corners, etc., assuming there is yielding in those regions? Are the results sensitive to mesh refinement in the same way that a linear-elastic analysis would be? Or does the use of a nonlinear material tend to be more 'forgiving' in the sense that it smooths over those large stress peaks?
My second question is along the same lines, but more related to strain. Clearly if we're happy to allow the material to yield, we then need to consider the failure criterion being excessive strain by checking that strains are within the capacity of the material. My question is similar: how sensitive are the strain values to the mesh refinement around notches and corners? I suppose I am mainly concerned with situations where tearing may occur in those locations. Note that I'm only concerned with monotonic/static loading, not really about impact, fatigue, etc.
I appreciate any advice in advance, references to books/papers would be really helpful, thanks all
I understand that when using linear-elastic analysis around sharp notches, re-entrant corners, and the like, there will always be a stress concentration that approaches infinite stress with increasing mesh refinement. I realise also that in a real structure, the geometry never really comes to a perfectly sharp corner if we 'zoom in' far enough, and in any case, the material will locally yield around those stress concentrations and tend to 'even out' the large stress peak predicted by the linear-elastic analysis.
I suppose my first question is this: if we use a nonlinear material in the FEA (e.g., assume an elastic-perfectly plastic stress-strain curve for steel), how do we then interpret the stresses around notches, re-entrant corners, etc., assuming there is yielding in those regions? Are the results sensitive to mesh refinement in the same way that a linear-elastic analysis would be? Or does the use of a nonlinear material tend to be more 'forgiving' in the sense that it smooths over those large stress peaks?
My second question is along the same lines, but more related to strain. Clearly if we're happy to allow the material to yield, we then need to consider the failure criterion being excessive strain by checking that strains are within the capacity of the material. My question is similar: how sensitive are the strain values to the mesh refinement around notches and corners? I suppose I am mainly concerned with situations where tearing may occur in those locations. Note that I'm only concerned with monotonic/static loading, not really about impact, fatigue, etc.
I appreciate any advice in advance, references to books/papers would be really helpful, thanks all