manstrom
Structural
- Jan 15, 2013
- 409
There have been a few posts on this, but I don't think this has been properly addressed by the ACI.
In the ACI 318-19, there is a new table for calculating one way shear. Table 22.5.5.1 shows three formulas.

Formula (a) and (b) simplify to the familiar equation phi*2*sqrt(f'c)*b*d times a new shape factor of lambda. This equation applies where Av min is met.
Formula (c) is the newer formula which applies where Av min is not provided. This utilizes rho. Assuming 0.0018 for rho, this simplifies to phi*1*sqrt(f'c)*b*d. Essentially half.
In previous versions of the code, there were exceptions to Av min for slabs, walls, wide beams etc. The intention is that Av min applies to beams, but walls and slabs are wider and can redistribute shear. My concern is that if I use formula (c) for one way shear (since I dont have stirrups in my walls), this is greatly impacting the design of isolated footings, laterally loaded basement walls, heavily loaded podium slabs.
In code revision summaries, they address the shape factor as a revision, but don't say "by the way we are cutting one way shear in half unless you put stirrups in your footings and walls"
So it Av min actually required in walls and footings? Table 9.6.3.1 is "Cases where Av Min is not required" which includes beams integral with a slab (but not a slab), shallow beams (but not walls) and wide beams (but not footings). The slab, wall and footing chapters refer directly to Chapter 22 and do not state a requirement for Av min for their respective elements.
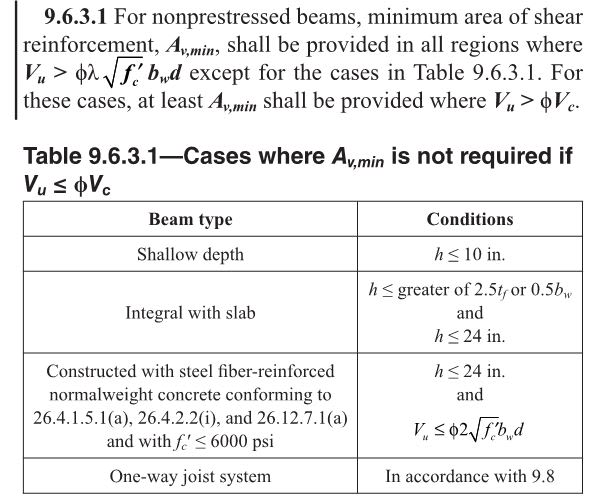
Even so, if Table 9.6.3.1 says Av min is not required, my design is penalized in Table 22.5.5.1 for having met Av min. The following Technical question seems to address this.
An ACI Technical question doesn't address walls and footings (I think they misunderstood the question)
A previous thread for reference.
Long winded. My question is - how are you interpreting the ACI 318-19 one way shear provisions for footings, walls, and heavily loaded slabs?
In the ACI 318-19, there is a new table for calculating one way shear. Table 22.5.5.1 shows three formulas.

Formula (a) and (b) simplify to the familiar equation phi*2*sqrt(f'c)*b*d times a new shape factor of lambda. This equation applies where Av min is met.
Formula (c) is the newer formula which applies where Av min is not provided. This utilizes rho. Assuming 0.0018 for rho, this simplifies to phi*1*sqrt(f'c)*b*d. Essentially half.
In previous versions of the code, there were exceptions to Av min for slabs, walls, wide beams etc. The intention is that Av min applies to beams, but walls and slabs are wider and can redistribute shear. My concern is that if I use formula (c) for one way shear (since I dont have stirrups in my walls), this is greatly impacting the design of isolated footings, laterally loaded basement walls, heavily loaded podium slabs.
In code revision summaries, they address the shape factor as a revision, but don't say "by the way we are cutting one way shear in half unless you put stirrups in your footings and walls"
So it Av min actually required in walls and footings? Table 9.6.3.1 is "Cases where Av Min is not required" which includes beams integral with a slab (but not a slab), shallow beams (but not walls) and wide beams (but not footings). The slab, wall and footing chapters refer directly to Chapter 22 and do not state a requirement for Av min for their respective elements.

Even so, if Table 9.6.3.1 says Av min is not required, my design is penalized in Table 22.5.5.1 for having met Av min. The following Technical question seems to address this.
An ACI Technical question doesn't address walls and footings (I think they misunderstood the question)
A previous thread for reference.
Long winded. My question is - how are you interpreting the ACI 318-19 one way shear provisions for footings, walls, and heavily loaded slabs?
