CARunderscore
Structural
- Nov 12, 2015
- 29
I suppose this should technically be in the Codes forum, but things look pretty dead over there, so...
As alluded to in this thread:
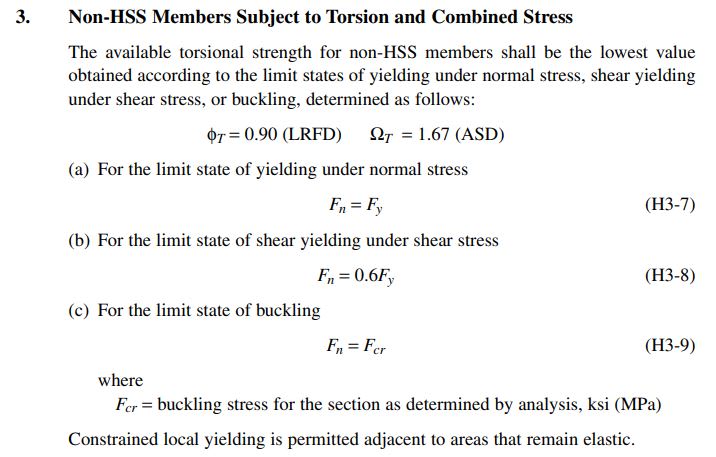
the Section H1 strength checks for doubly-symmetric shapes in flexure (and, therefore, flexure in combination with other stresses) of steel members in AISC 360-16 - Specification for Structural Steel Buildings are expressed as functions of the plastic section modulus instead of elastic section modulus, at least as long as buckling does not control. However, the methods of Section H3 for checking torsion are stress-based, and the maximum normal stress allowed by Equation H3-7 for combined normal stresses due to axial force, biaxial bending, AND torsion is lower than the stress allowed by Section H1 for axial force and bending only.
I can't imagine the intent is for a beam with negligible or zero torsion to "fail" a torsion stress check while passing the combined axial/flexure check, so I'm thinking that one of the following is the case:
1. The language in Section H1 regarding "members constrained to bend about a geometric axis" is meant to preclude situations where appreciable torsion is possible. But even then, Equation H2-1 for "other" members is a function of plastic section modulus for some sections.
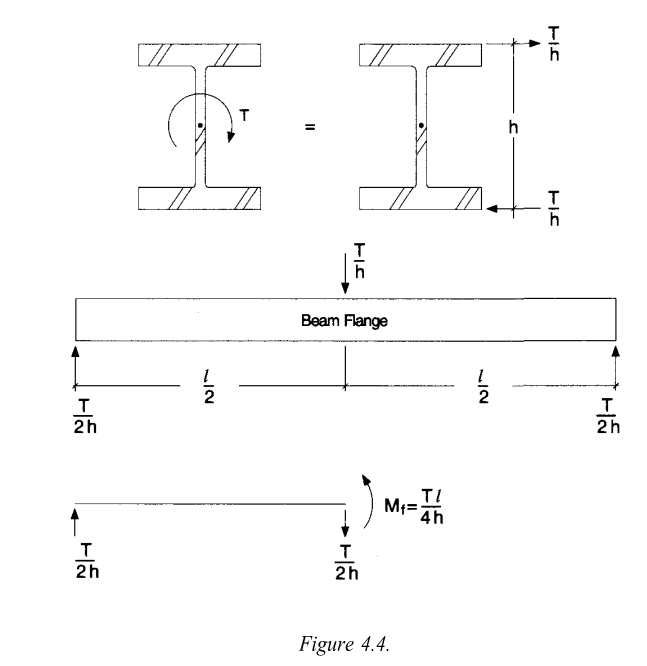
2. The clause in Section H3 "Constrained local yielding is permitted adjacent to areas that remain elastic" is meant to permit localized yielding at the flange tips under strength-level loads. But AISC Design Guide 9 (referenced but not adopted as part of AISC 360) pretty explicitly uses elastic section modulus for strength-level checks.
Thoughts?
As alluded to in this thread:
the Section H1 strength checks for doubly-symmetric shapes in flexure (and, therefore, flexure in combination with other stresses) of steel members in AISC 360-16 - Specification for Structural Steel Buildings are expressed as functions of the plastic section modulus instead of elastic section modulus, at least as long as buckling does not control. However, the methods of Section H3 for checking torsion are stress-based, and the maximum normal stress allowed by Equation H3-7 for combined normal stresses due to axial force, biaxial bending, AND torsion is lower than the stress allowed by Section H1 for axial force and bending only.
I can't imagine the intent is for a beam with negligible or zero torsion to "fail" a torsion stress check while passing the combined axial/flexure check, so I'm thinking that one of the following is the case:
1. The language in Section H1 regarding "members constrained to bend about a geometric axis" is meant to preclude situations where appreciable torsion is possible. But even then, Equation H2-1 for "other" members is a function of plastic section modulus for some sections.
2. The clause in Section H3 "Constrained local yielding is permitted adjacent to areas that remain elastic" is meant to permit localized yielding at the flange tips under strength-level loads. But AISC Design Guide 9 (referenced but not adopted as part of AISC 360) pretty explicitly uses elastic section modulus for strength-level checks.
Thoughts?