Are minimum reinforcements additive? 6

[darkjmf1]

Hello,
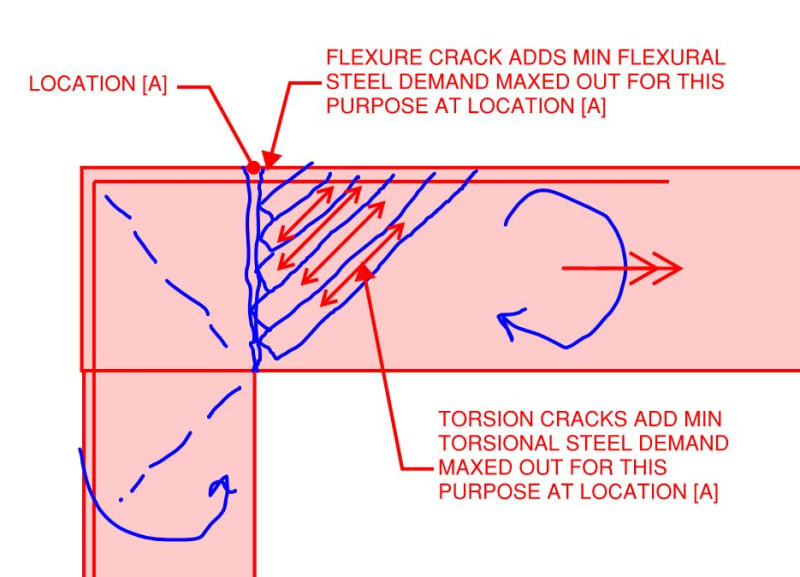
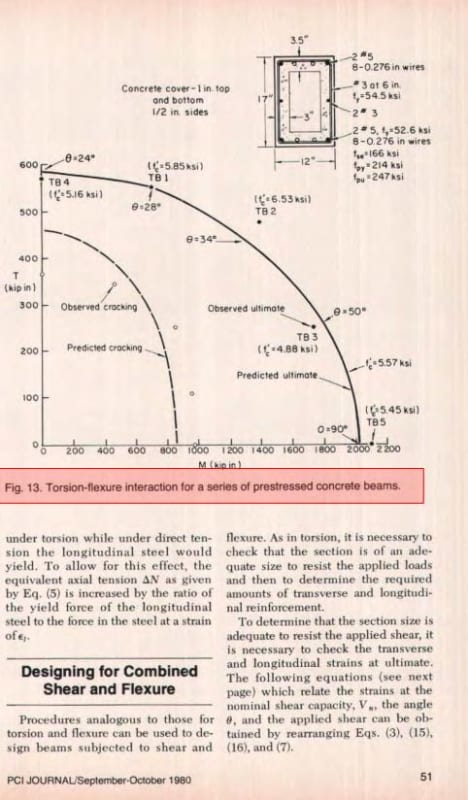
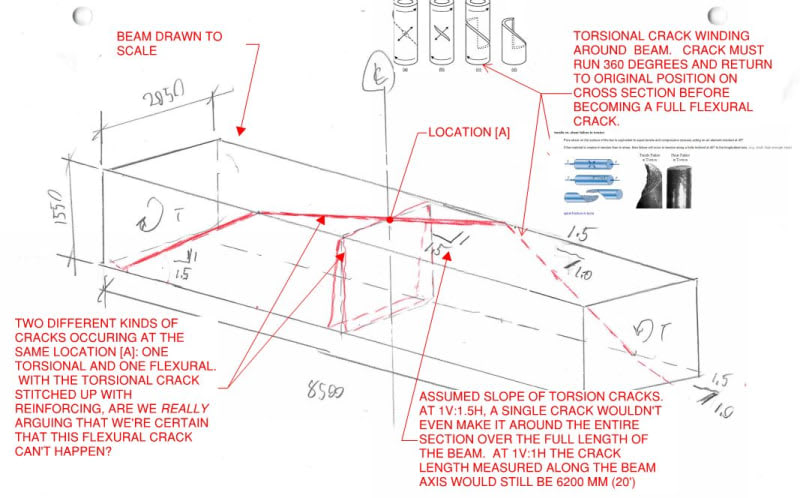
I am designing a RC beam with a section which, due to architectonical reasons, is much larger than required, resulting in minimum reinforcement both for bending and torsion.
Do I have to calculate both As,min for bending and longitudinal Asl,min for torsion separately and eventually adding them up in the section?
Or could I just calculate both As and Asl required by analysis and just check if the sum of them complies with As,min and Asl,min?
I am using ACI, but I guess this discussion could be applicable for any concrete code.
Thank you all in advance.

 

[KootK]

Not sure that you understood my argument. I'm not re-distributing torsion. I'm re-distributing beam end moments that were the cause of torsion in their supporting girder.

Clearly I did not understand. But, in my defense, you didn't give me much of a chance given your specific wording:

once the the beam cracks for torsion (and presumably re-distributes it's torque towards the center of the beam)

The end moment of a fixed end beam framing into a girder causes a torque in the girder, right? When the cracking occurs (flex cracking in beam, or torsional cracking in girder) that fixed end moment gets re-distributed towards the center of the beam. Therefore, the girder no longer experiences the torque.... because the beams end moment has been relieved / redistributed away from the girder.

That's all kosher. It's just not what you wrote originally, hence my concern.

 

[Tomfh]

The code writers aren't super-conservative with these minimum reinforcement quantities.

This is something I often wonder about. How many beams with minimum reinforcement would actually be ductile when the real cracking moment is reached. Are the code equations empirical? Or are they merely theoretical based on assumed concrete tensile strength?

 

[Tomfh]

From the 4th post, that gives 0.62*10 bars for flexure and 0.62*11 for torsion = 13 bars as an estimate of minimum consistent with the requirements for pure flexure/torsion.

I think it will crack slightly before that, but assuming it is 62%, only half the torsion bars are in the flexure tensile face, so 0.62*10 + 0.5 * 0.62 * 11 = 9.6 for that face, ie less than flexural minimum.

Can anyone come up with an example of combined flexure and torsion forces which are on the point of cracking the concrete, but whose longitudinal stresses at any given point would not be dealt with by the flexural and torsional minimums?

 

[JoshPlumSE]

Clearly I did not understand. But, in my defense, you didn't give me much of a chance given your specific wording

Egad!! You mean other engineers can't perfectly interpret my meaning when my post is worded poorly?

 

[KootK]

Egad!! You mean other engineers can't perfectly interpret my meaning when my post is worded poorly? wink

I feel you brother. A half dozen attempts in and Tomfh still thinks that I'm trying to double count concrete tension when, in fact, I'm trying to say almost the reverse (excess, combined demand). I just can't find the right combination of words or the right sketch to turn it around.

 

[KootK]

How many beams with minimum reinforcement would actually be ductile when the real cracking moment is reached?

Among the designs where the minimums governed, probably next to none of them. I see this as a fun theoretical thing but, frankly, of little practical value. I'd be pleased as punch if the code ditched all this stuff and just said:

1) Though shalt provide [A%] longitudinal reinforcing in the flexural tension face all beams and;
2) [B%] longitudinal reinforcing in the remaining faces of all beams.

THE END.

This can be a bit conservative, nobody's going to give a damn. And this is something that almost certainly would be better done empirically if it is not already. In reality, I just consider the minimums to be little more guides to designing marginally reinforced things anyhow. And, as seems to be the case here, getting all fancy with it produces odd results in some situations.

... if I've done Mohr's circle correctly.

That was awesome, thanks. Some additional thoughts that I have on that:

1) Imagine that one were to take a beam with no cracks of any kind and study when the first flexural crack would appear. Here, I'm talking about a conventional, orthogonal flexural crack at the point of maximum moment. Would the presence of torsional stresses cause that crack to open up at a lower induced moment? You betcha. And vice versa.

2) I've not been considering #1 because I see thing unfolding as follows:

a) You add some load, both torsion and bending. You've got interacting stresses all over but nothing's cracked yet.

b) Yuu add some load and, maybe, the torsion crack forms first ahead of the governing flexural crack. Obviously, it's improbable that the governing flexural crack and the governing torsional crack will form simultaneously.

c) Now, because of the torsional crack, elastic torsional stresses are substantially relieved and there is little to no torsional stress at the tensile tip of the governing flexural crack.

d) You're back to having to deal with the regular flexural Mcr rather than a reduced flexural Mcr that one would expect to accompany a concurrent, torsional stress field. This is how I see thing but I will shortly post something to the contrary.

 

[KootK]

Can anyone come up with an example of combined flexure and torsion forces which are on the point of cracking the concrete, but whose longitudinal stresses at any given point would not be dealt with by the flexural and torsional minimums?

Yeah, I can. But:

1) All of this stuff is about what happens just after cracking so I'm going with that as my starting point.

2) Obviously, this is going to reflect my view of things which you dispute.

 

[Tomfh]

Yeah, I can.

Please do so then. A precracked member with combined flexural and torsional tensile stresses in a face that would not be captured by minimum flexural tensile reinforcement in that face.

 

[KootK]

So I had to dig deep but I managed to find a published reference that deals specifically with the combination of the minimum requirements for torsion and flexure. That reference is attached. Below, I've highlighted the bits that I believe are of particular relevance. Some things to note about the reference:

1) I believe that it's public domain.

2) It's crazy old and may well be superseded by other stuff.

3) It's by Michael Collins who is, for all intents and purposes, the Jesus of modern concrete shear design.

4) Per usual with Collins, all of the good stuff flows from the Modified Compression Field Theory of shear.

If one suspends disbelief and takes the Collins reference as gospel, I believe that the following conclusions will be manifest:

A) The minimum requirements for flexure and torsion are indeed additive, at least to a degree.

B) The minimums need not be added at their full, individual values (reference uses "pure" values for this).

In my opinion, the suggested procedure is a bit onerous for routine design. I speculate that we may have the ACI provisions that we do because the code writers thought to themselves:

- designing for one min or the other would seem to be insufficient.
- designing for the interaction is kinda hard.
- screw it, lets add them at their full, pure values and let it be a bit overkill.

 

[KootK]

Please do so then. A precracked member with combined flexural and torsional tensile stresses in a face that would not be captured by minimum flexural tensile reinforcement in that face.

Already did, prior to your posting this. Unfortunately, this can't be discussed meaningfully util just after the concrete cracks. It is that cracking, after all, that creates the tensile demand in the longitudinal reinforcing.

 

[KootK]

I assume it is because these elements are not so prone to being torsioned, but the fact is that I also have in my project columns in such situation. How should I design their torsional reinforcement? Just deploying the bars required by calculation, without a minimum?

1) If your columns are really seeing that much torsion, I'd revisit your model and/or the lateral stiffness of your building. That, unless these columns are freestanding, cantilevered sign supports or something exotic like that.

2) Once a column has attracted enough torsion for me to care, I'd be calling that column a "beam-column" and applying the beam minimums for torsion. As the designer, the onus is really on you to assess the situation critically and decide which code provisions make sense. Nobody's foreseeing that you'll ignore certain load actions just because a particular element bears a conventional label in the industry dogma.

3) Another reason for columns not to be heavily impacted by the minimum longitudinal requirement is that any reliable dead load on the column effectively performs that same function, as though the column were prestressed. Moreover, any precompression will increase Tcr.

 

[Tomfh]

Already did, prior to your posting this.

No, that doesn’t actually answer it.

 

[KootK]

No, that doesn’t actually answer it.

How could it given that it's an impossible question taken at face value? Discussing the rebar demand in an uncracked member is pointless.

 

[Celt83]

Here is how I look at it, If you have only provided the absolute minimum amount of steel then for flexure lets say you have (3)#7's bottom, bar in each corner and mid line, the minimum provision is setup up so that at ultimate strength the allowable moment exceeds the cracking moment to ensure a ductile failure mode. In my admittedly limited runs on cross sections As,min yields a steel strain of around 0.04 ish as such the steel has yielded so you achieve T = As*Fy, ignoring strain hardening, this is the limit strength of the steel the total Tension in the (3)#7 bars is set up to be in equilibrium with the flexural compression block, ie the tension from this steel is now used up and the cross section is in equilibrium. Adding torsion to the same cross section location into the mix now introduces another set of compression forces in the cross section which require a sub set of steel Tension forces to regain equilibrium. Since I'm already accounting for As*Fy in my flexural steel I now need to add additional steel to allow me to maintain the flexural strength I had, otherwise we'd be utilizing less steel tension for straight flexure creating a higher neutral axis, less flexural compression and thus a lower ultimate flexural moment capacity.

Open Source Structural Applications:

https://github.com/buddyd16/Structural-Engineering

 

[Tomfh]

Unfortunately, this can't be discussed meaningfully util just after the concrete cracks.

How could it given that it's an impossible question taken at face value?

I was discussing conditions just "prior to cracking" as that was your original premise. That's the point which defines minimum steel requirements.

 

[Settingsun]

If one suspends disbelief and takes the Collins reference as gospel, I believe that the following conclusions will be manifest:

A) The minimum requirements for flexure and torsion are indeed additive, at least to a degree.

B) The minimums need not be added at their full, individual values (reference uses "pure" values for this).

In my opinion, the suggested procedure is a bit onerous for routine design. I speculate that we may have the ACI provisions that we do because the code writers thought to themselves:

- designing for one min or the other would seem to be insufficient.
- designing for the interaction is kinda hard.
- screw it, lets add them at their full, pure values and let it be a bit overkill.

Collins & Mitchell called for 1.2*cracking load as minimum reinforcement. For pure flexure, I think the ACI requirement is more than this. For a beam of f'c = 40 MPa (5800 psi), fy = 500 MPa (72,500 psi), d = 0.87*D, 1.2*Mcr requires about 0.21% reinforcement. ACI 318-14 requires 0.28% (requirement 9.6.1.2(b) governs). Whether interaction with shear/torsion is the reason for the difference or not, it's probably covered by treating them as separate requirements rather than additive.

 

[GC_Hopi]

What if the torsion crack is at the bottom of the beam at location A? Would torsion and flexural be addative?

 

[GC_Hopi]

So does the torsional force have a component along the beam axis and perpendicular to the beam axis?

 

[Tomfh]

Yes the torsional force has a longitudinal (tension) component, and a transverse tension component. The net result is diagonal tension pattern spiralling around the beam, hence the spiral fracture pattern.

In the example above the beam would actually crack at the bottom, where the flexural tensile force adds to the torsional tensile force. You’d get a combined flexure torsion crack.