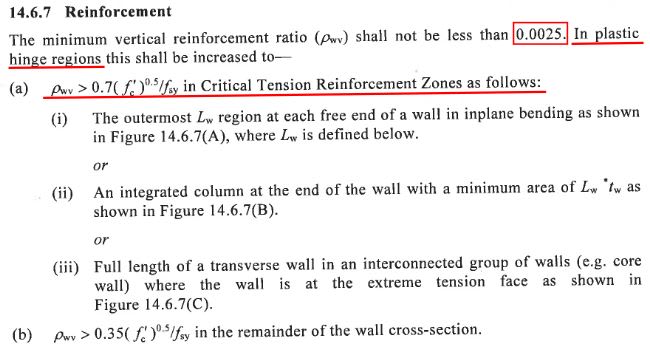
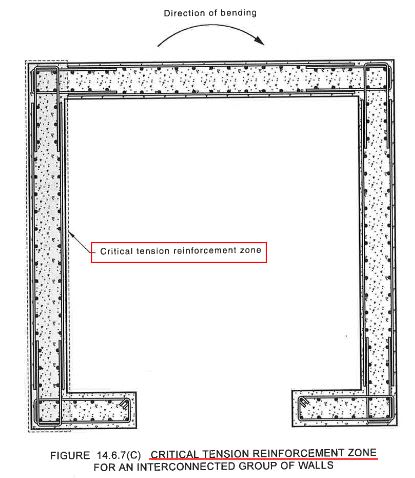
Aside from all the closed ligs, the new code's minimum reinforcement requirements for EQ also appear to have changed/increased:
We have a core box like this, with many internal walls:
The core is mainly in compression, with low tension forces, especially for the EQ cases. The wind cases do generate some tensions but they are still small.
I've been trying to find a way to justify that given the low tensions, there is no real "plastic hinge region", however, based on my reading online, plastic hinge zones can be defined regardless of whether the concrete element is in tension or compression.
I feel like I'm missing something though as the core will require N32-150 for the first 10 floors due to the core walls being thick. It doesn't make sense to have such heavy reinforcements given the tensions are so small.
Looking forward to the discussions!

We have a core box like this, with many internal walls:

The core is mainly in compression, with low tension forces, especially for the EQ cases. The wind cases do generate some tensions but they are still small.
I've been trying to find a way to justify that given the low tensions, there is no real "plastic hinge region", however, based on my reading online, plastic hinge zones can be defined regardless of whether the concrete element is in tension or compression.
I feel like I'm missing something though as the core will require N32-150 for the first 10 floors due to the core walls being thick. It doesn't make sense to have such heavy reinforcements given the tensions are so small.
Looking forward to the discussions!
