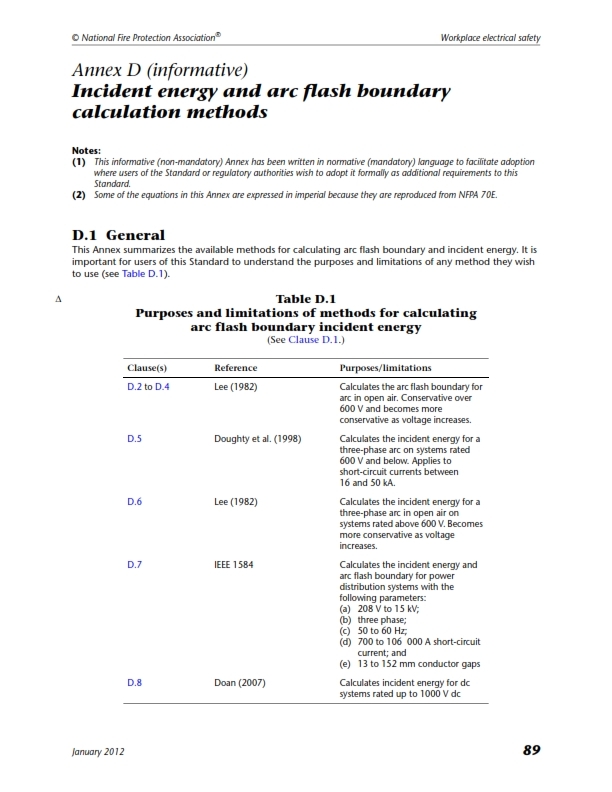
Hello
I am conducting an arc flash study of a power distribution system using IEEE 1584. For calculating the arcing current, I need to determine what's the available bolted three phase short circuit current. How is this determined? Is it the utility that provides this parameter? Does this value change at each bus level (from upstream to downstream)?
I am conducting an arc flash study of a power distribution system using IEEE 1584. For calculating the arcing current, I need to determine what's the available bolted three phase short circuit current. How is this determined? Is it the utility that provides this parameter? Does this value change at each bus level (from upstream to downstream)?