PSR_1
Civil/Environmental
- Aug 9, 2016
- 56
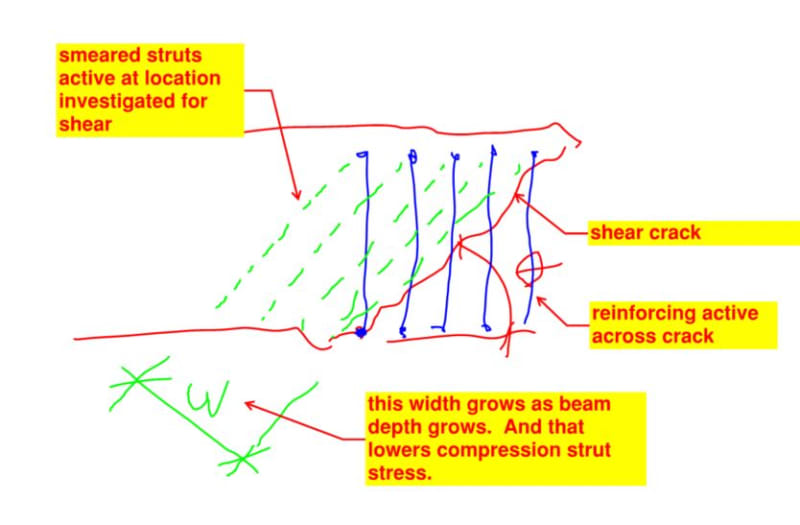
Most codes cover the case of beams deficient in shear reinforcement only and usually they propose CFRP to add to the shear strength of a beam on top of shear rebar and concrete. What would be the response of a beam which has a shear force that is more than Vrd,max? usually this case is solved by jacketing the beam to increase it's depth, is there a CFRP equivalent solution?