Keithkingdon1st
Mechanical
Hello everyone,
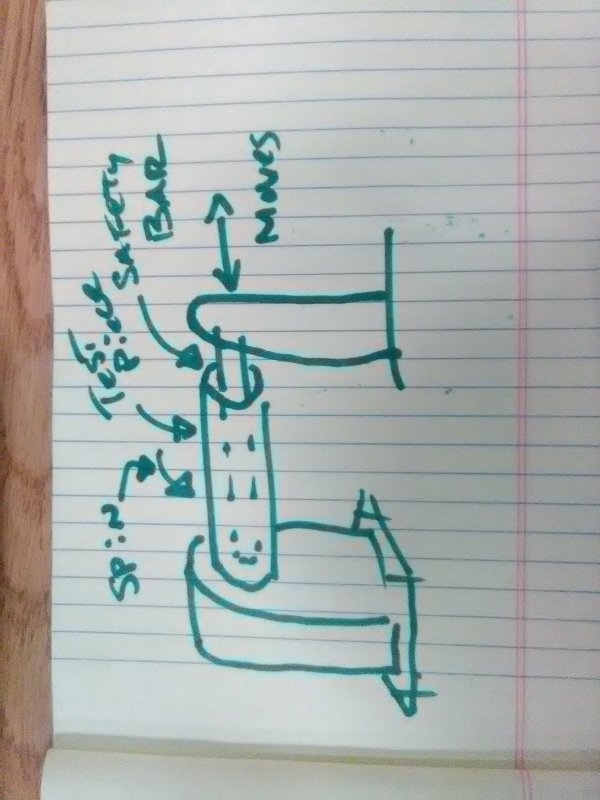
I have a problem that I was asked. The company that I work for is building a fatigue testing machine, they asked me to calculate a component of said machine. The part I am calculating is the safety leash/lasso that would keep the shaft from destroying the machine in the event that the shaft breaks in half.
So the problem is; a 40000 lb. perpendicular force is applied to the end of an initially stationary 6 foot section with the other end attached to a pivot point. The section is a hollow mild steel tube with OD 9.625in and ID 8.8.35in. I must find the force required to stop the shaft when it rotates 90degrees neglecting gravity.
I can’t seem to figure out this problem. Converting to SI I get the following:
Moment applied of the end = 325396.308 Nm
Mass of section = 88 kg
Radius of Gyration = 0.08296 m
Moment of Inertia = 0.6056 kgm2
Angular Acceleration = 537312.26 rad/sec2
Angular Velocity at 90 degrees = 537.31 rad/sec
Angular Momentum = 325.396 kgm2/sec
Force required to stop = Original 40000lbs
The supervisor says he would like the shaft to go 0 degrees to 90 degrees in about 1ms
The equations I used are:
Torque = Force x Distance
Inertia = Mass x Radius of Gyration
Radius of Gyration = ((D2+d2)1/2)/4
Angular Acceleration = Torque/Inertia
Angular Velocity = Angular Acceleration x Time
Angular Momentum = Inertia x Angular Velocity
I have tried to solve the problem many times but have gotten nowhere. Any advice on where I am making a mistake? Would the force required to stop the shaft be the initial 40000 lbs put into the system?
Thanks
I have a problem that I was asked. The company that I work for is building a fatigue testing machine, they asked me to calculate a component of said machine. The part I am calculating is the safety leash/lasso that would keep the shaft from destroying the machine in the event that the shaft breaks in half.
So the problem is; a 40000 lb. perpendicular force is applied to the end of an initially stationary 6 foot section with the other end attached to a pivot point. The section is a hollow mild steel tube with OD 9.625in and ID 8.8.35in. I must find the force required to stop the shaft when it rotates 90degrees neglecting gravity.
I can’t seem to figure out this problem. Converting to SI I get the following:
Moment applied of the end = 325396.308 Nm
Mass of section = 88 kg
Radius of Gyration = 0.08296 m
Moment of Inertia = 0.6056 kgm2
Angular Acceleration = 537312.26 rad/sec2
Angular Velocity at 90 degrees = 537.31 rad/sec
Angular Momentum = 325.396 kgm2/sec
Force required to stop = Original 40000lbs
The supervisor says he would like the shaft to go 0 degrees to 90 degrees in about 1ms
The equations I used are:
Torque = Force x Distance
Inertia = Mass x Radius of Gyration
Radius of Gyration = ((D2+d2)1/2)/4
Angular Acceleration = Torque/Inertia
Angular Velocity = Angular Acceleration x Time
Angular Momentum = Inertia x Angular Velocity
I have tried to solve the problem many times but have gotten nowhere. Any advice on where I am making a mistake? Would the force required to stop the shaft be the initial 40000 lbs put into the system?
Thanks