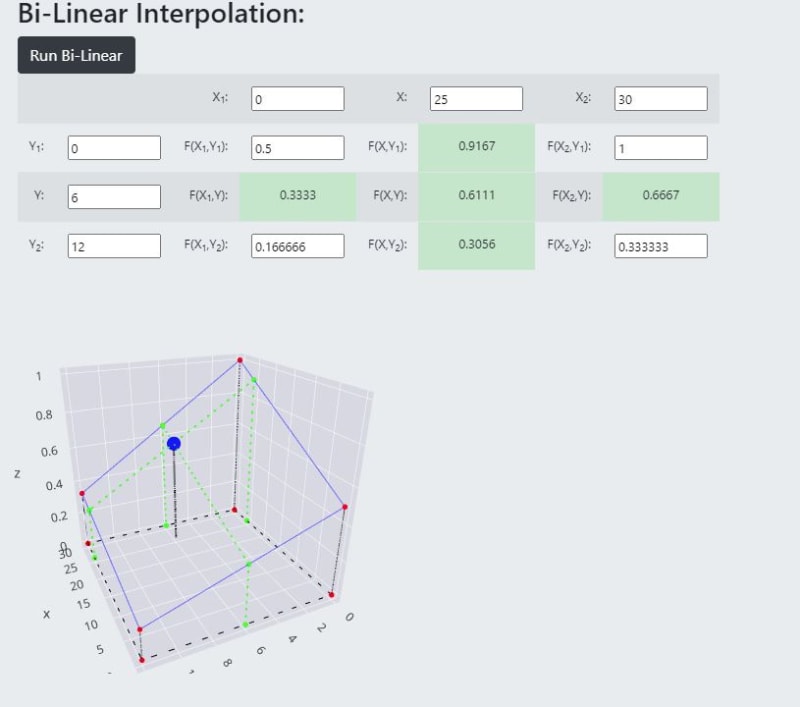
I am trying to get the deflection D(x) under a combined foundation.
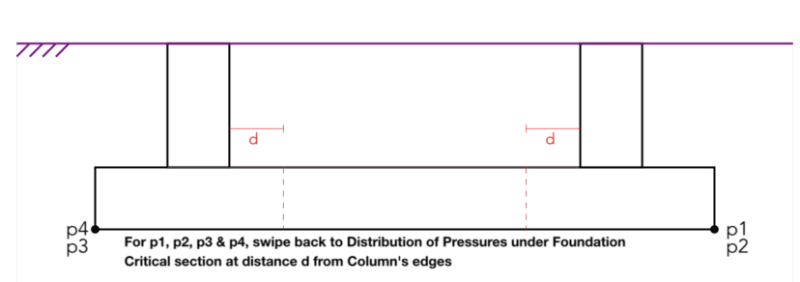
I manage to get the function bending moment M(x) by dividing the area in 3 places.
- Equation 1: from Edge of foundation to First Column C1
- Equation 2: From Column C1 to Column C2
- Equation 3: From Column C2 to the edge of foundation
by integrating the differential equation of the deflection Curve, I got the function deflection(x) but with 6 unknowns parameters (Const1, Const2, Const3, Const4, Const4, Const6)
in order to solve these unknown const, I should have 6 equations.
But what I have is just 2 equations.
the First one: Deflection from Equation 1 at C1 = Deflection from Equation 2 at C1
Second one: Deflection from equation 2 at column C2 = Deflection from equation 3 at column C2.
how to get the remaining 4 equations.
I cannot consider the deflection at column 1 and column 2 = 0 cause underneath is soil.
any advise or help so I can proceed cause I already stuck for almost 1 week.

I manage to get the function bending moment M(x) by dividing the area in 3 places.
- Equation 1: from Edge of foundation to First Column C1
- Equation 2: From Column C1 to Column C2
- Equation 3: From Column C2 to the edge of foundation
by integrating the differential equation of the deflection Curve, I got the function deflection(x) but with 6 unknowns parameters (Const1, Const2, Const3, Const4, Const4, Const6)
in order to solve these unknown const, I should have 6 equations.
But what I have is just 2 equations.
the First one: Deflection from Equation 1 at C1 = Deflection from Equation 2 at C1
Second one: Deflection from equation 2 at column C2 = Deflection from equation 3 at column C2.
how to get the remaining 4 equations.
I cannot consider the deflection at column 1 and column 2 = 0 cause underneath is soil.
any advise or help so I can proceed cause I already stuck for almost 1 week.