StructuralDesigner91
Structural
- Jul 23, 2019
- 4
Hi,
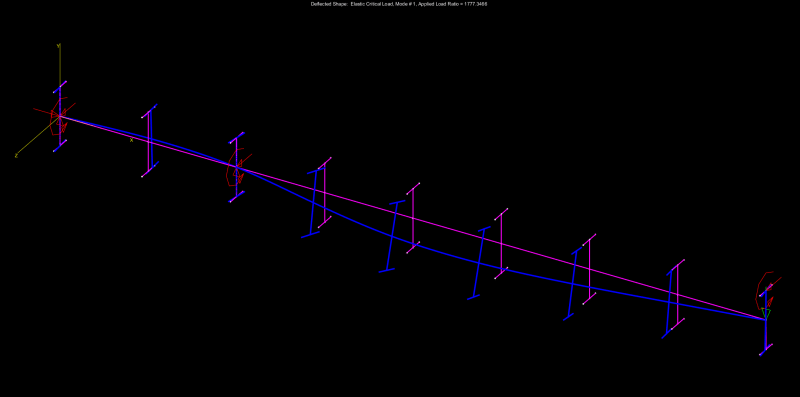
I'm working on a temporary beam that will cantilever 20 meters, I'm looking for information regarding the effective length to use. Most publication only discuss the restraint condition at the tip and the root, but what effects do adding intermediate bracing have on the effective length. Its a built up beam 2500mm deep. I've read Galambos guide to stability design criteria for metal structures but there is no discussion regarding the effect of intermediate stiffeners.
Any guidance or information is appreciated.
I'm working on a temporary beam that will cantilever 20 meters, I'm looking for information regarding the effective length to use. Most publication only discuss the restraint condition at the tip and the root, but what effects do adding intermediate bracing have on the effective length. Its a built up beam 2500mm deep. I've read Galambos guide to stability design criteria for metal structures but there is no discussion regarding the effect of intermediate stiffeners.
Any guidance or information is appreciated.

![[wink] [wink] [wink]](/data/assets/smilies/wink.gif)

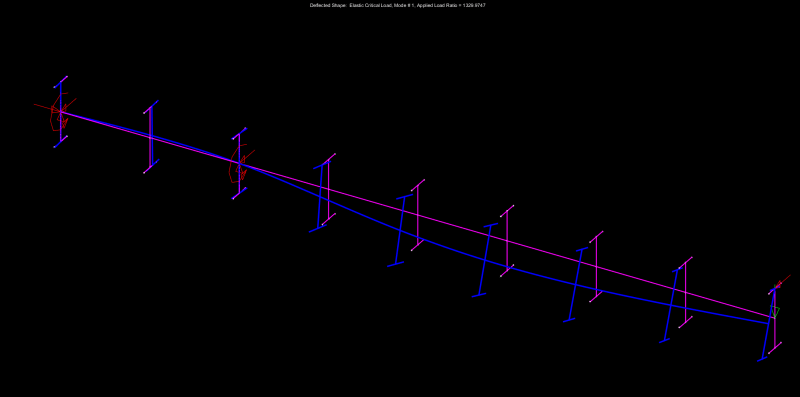
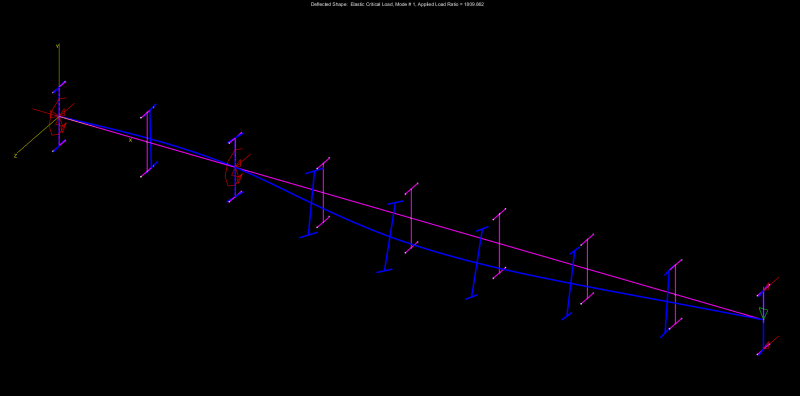
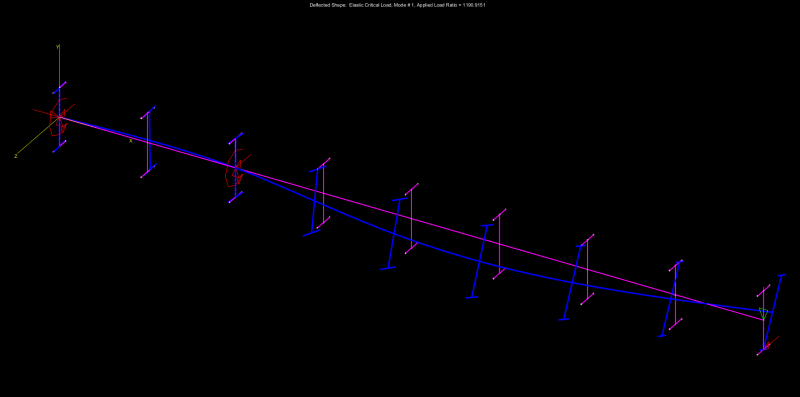
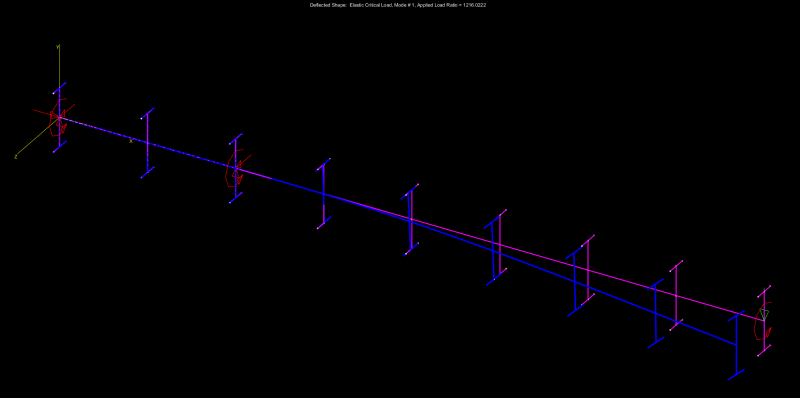
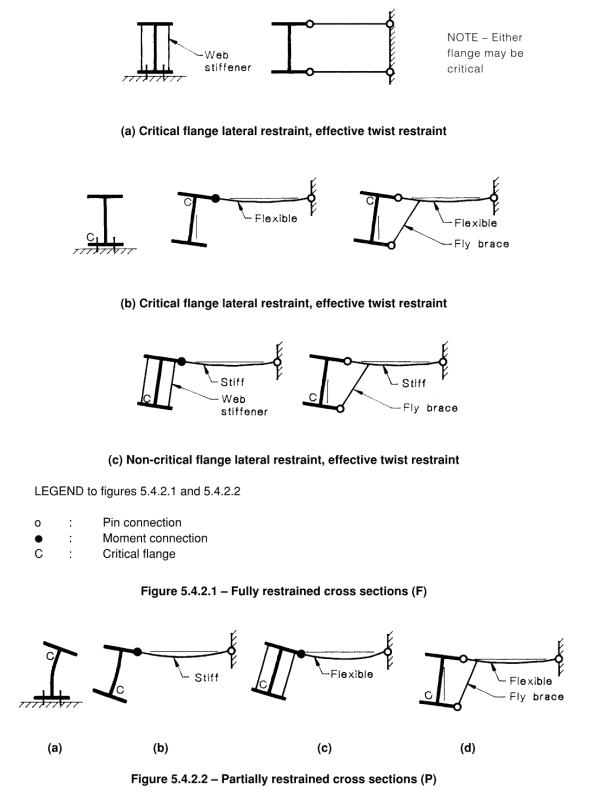

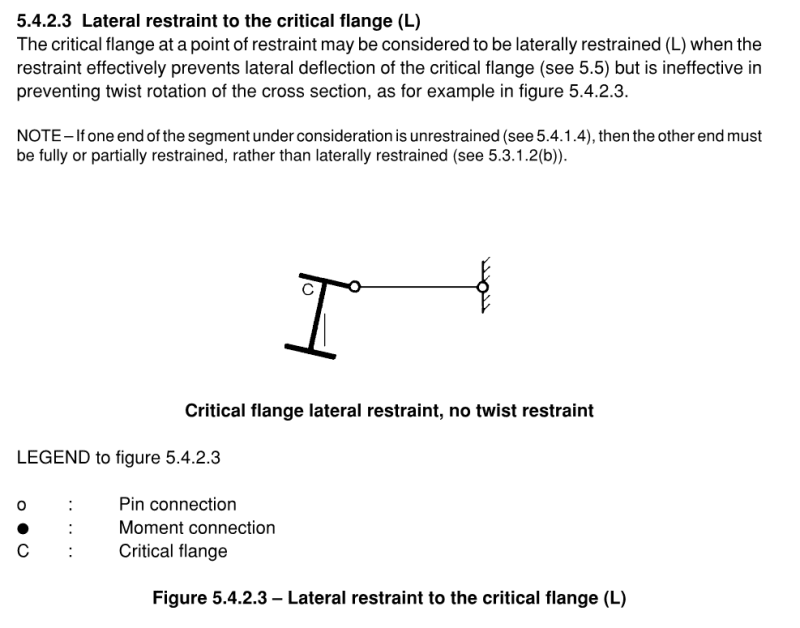
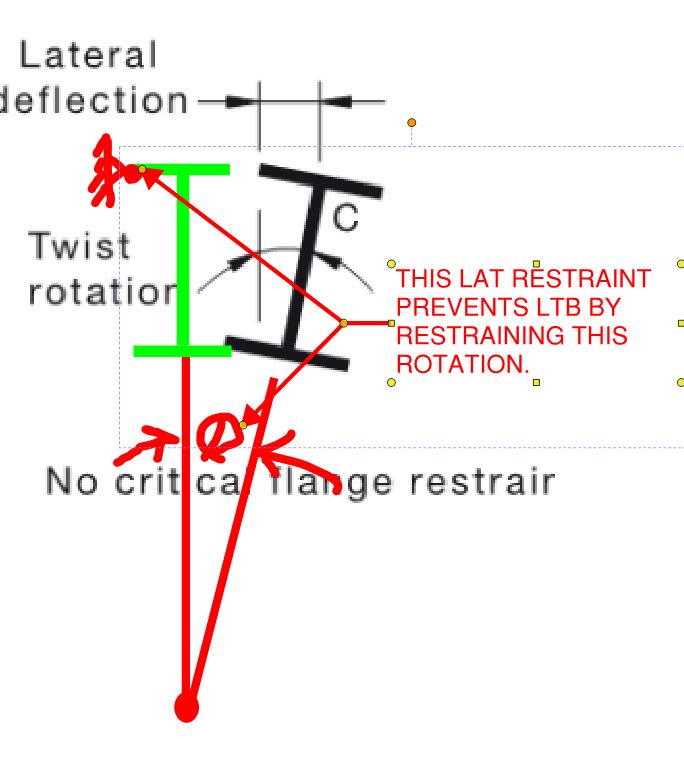
![[2thumbsup] [2thumbsup] [2thumbsup]](/data/assets/smilies/2thumbsup.gif) .
.