Force against a gate in a closed conduit

[Jake-Designer]

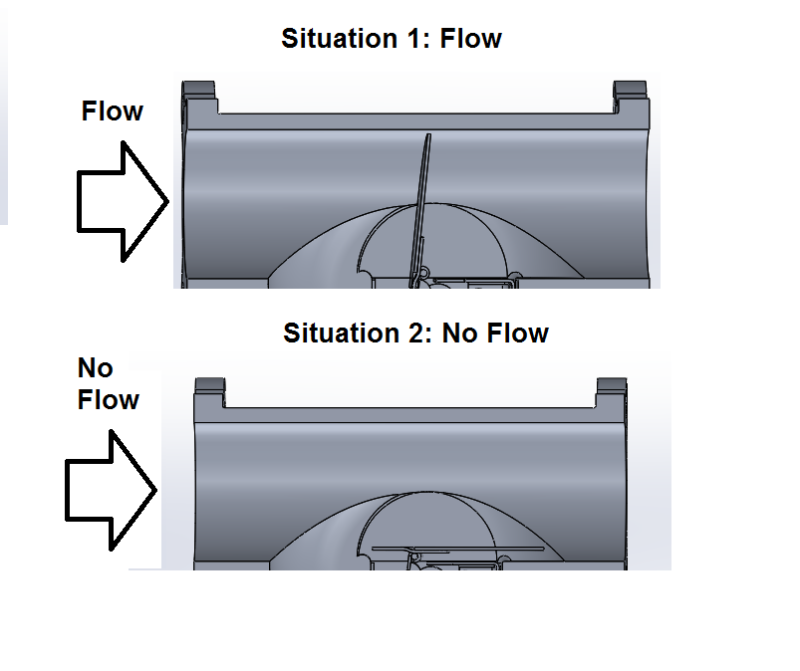
I am working on a design problem where I have a pipe filled with water. Within a pipe there is a gate connected to a hinge. I am trying to gain a better understanding of two situations. The first situation is when a boost pump moves the fluid what is the force against the gate, I believe I can model with dynamic pressure where F = q * Area and q = (1/2) * density * velocity * velocity. The second situation is if the boost pump is turned off, stopping the flow of fluid and I apply a moment to the hinge what factors do I need to consider for returning it back to the upright position.

I have read through some online resources and some fluid mechanics textbooks that I have but haven't been able to find a concrete example of what I am doing. Any good references would be greatly appreciated.

Thanks!

 

[bimr]

If the fluid is not moving, then there is no pressure across the gate.

If the fluid is not moving (and hydrostatic pressure is holding the gate closed) then the force is the static head (water column) * the area of the gate.

https://mysite.du.edu/~jcalvert/tech/fluids/hydstat.htm

BigInch, thanks for the correction.

 

[BigInch]

That's a triangular pressure load, so Max Pressure is [ρ]gh at the base of the gate, but the force on the gate itself (applied at 2*h/3 below the water line) is 1/2 [ρ]gh[sup]2[/sup] * Gate_Width

Find what you like to do, earn a living at it, and then make your lifestyle fit your income. — Chuck Yeager

 

[25362]

(2/3)h is the centroid of the right triangle of forces.

 

[BigInch]

Welcome.
Now the dynamic component ...
Pump running and flow velocity hitting a closed gate
Dynamic pressure of fluid hitting a stop (stagnation pressure) is 1/2 * [ρ]V[lsup]2[/sup]
You see that in the dynamic term of the Bernoulli equation.

https://www.princeton.edu/~asmits/Bicycle_web/Bernoulli.html

Find what you like to do, earn a living at it, and then make your lifestyle fit your income. — Chuck Yeager

 

[dbill74]

A sketch or picture of your situation would be most useful.

 

[Jake-Designer]

Thank you for the replies. Here is a sketch of the problem.

In both cases the pipe is completely filled with water. You can see the first image with the gate upright and a boost pump provides flow causing the gate to rotate about the hinge. The second image is when the boost pump is shut down and flow has stopped. What moment would I need to apply to move the gate back to the upright position.

Bimr and BigInch this is initially how I was looking at the problem using the pressure distribution due to the weight of the fluid at the centroid. But how does the situation get effected by not having a free surface? Being in a closed conduit. Most of the problems I have seen are the situation of a free surface.

BigInch this is how I was looking at the dynamic portion as well. I was looking at perhaps drag becoming a factor depending on fluid velocity, but the dynamic pressure seemed to be giving me close results to what I am experiencing.

 

[IRstuff]

Seems to me that your 2nd case is dependent on the speed at which you want to close the gate. If you were to move it slowly, there would be time for the fluid to move around the top edge of the gate, and the force would be relatively low.

TTFN (ta ta for now)
I can do absolutely anything. I'm an expert!

https://www.youtube.com/watch?v=BKorP55Aqvg

faq731-376 forum1529 Entire Forum list

http://www.eng-tips.com/forumlist.cfm

 

[BigInch]

For a closed conduit the initial pressure at the top of the gate Po is equal to the internal pipe pressure at that same point. From there the pressure increases by pgh as you move down to the bottom of the gate.

Dynamic pressure starts out equal to "drag", except then it gets a little fancier by multiplying 1/2 pV^2 by a "shape factor". A shape factor for a rectangle in a free flow stream (a billboard in the wind) is around 1.4

Technology is stealing American jobs. Stop H1-Bs for robots.

 

[Jake-Designer]

IRstuff

I want to make sure I understand all the forces involved and ensure the moment I apply (which I am designing a torsion spring but I just left it as a general moment for simplicity in this discussion) is enough to overcome them when it is in the "down" position.

BigInch
Can you clarify what you mean by top of the gate and bottom of the gate? This would be the situation where it is standing up? In the case where it is "down" (I have attached that picture, let me know if I explained it well) would the only forces be due to the static pressure due to the height of the column above the gate or being in a pipe would internal pressure within the water in the pipe be added to this.

 

[BigInch]

Yes, when it is standing up. When it is laying down, pressure is the same all over the gate, on the top and the bottom side of it as well, thus net unbalanced force is 0.



Technology is stealing American jobs. Stop H1-Bs for robots.

 

[Jake-Designer]

Thanks for the replies. I think I understand now. In the case where the gate is in the down position with no flow I was trying to use the hydrostatic force applied on the top of the gate neglecting the hydrostatic force on the bottom of that gate. As far as for net forces the only forces to account for in returning the gate back to the upright position would be the downward force due to the weight of the gate, the buoyant force due to displaced volume and frictional forces at the hinge.

 

[BigInch]

Correct, as long as there is no flow impinging on the gate as it is going up.

Technology is stealing American jobs. Stop H1-Bs for robots.