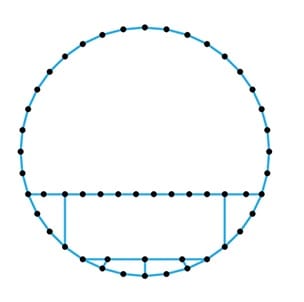
We had a discussion in our group on how do the hoop stresses are calculated in a fuselage section composed of different radii (non circular shape).
Do you use the biggest radius (being conservative)?
Do you use the local radius for a given panel?
Do you use the opposite radius of the panel you are calculating?
Do you use an average of radii?
Which method is the most broad and complete one that we could rely on and give away the conservatism when needed.
Do you know any methodology or a paper with a methodology?
Thanks in advance for any feedback.
Do you use the biggest radius (being conservative)?
Do you use the local radius for a given panel?
Do you use the opposite radius of the panel you are calculating?
Do you use an average of radii?
Which method is the most broad and complete one that we could rely on and give away the conservatism when needed.
Do you know any methodology or a paper with a methodology?
Thanks in advance for any feedback.