ITsSB
Structural
- May 6, 2022
- 34
I don't know if this belongs here but,
I want to create a shell structure created by three half circles vertically rotated at 60°. (see attached image). So, is there any easy way/tool to create this meshed shell? (I will use it elsewhere for complete structural analysis.) So far I have tried using GMSH but couldn't generate the mesh/surface.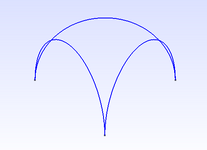
I want to create a shell structure created by three half circles vertically rotated at 60°. (see attached image). So, is there any easy way/tool to create this meshed shell? (I will use it elsewhere for complete structural analysis.) So far I have tried using GMSH but couldn't generate the mesh/surface.

Last edited:
