Davide Recchia
Mechanical
- Jun 3, 2019
- 30
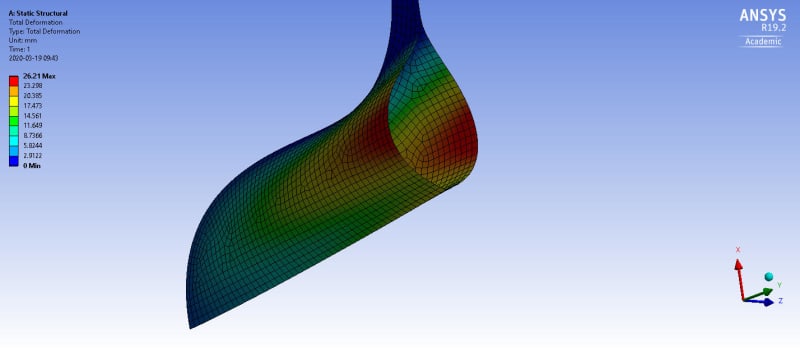
How would I calculate the maximum possible inflated volume of an initially flat sealed bag which has an irregular shape (two examples below) made out of two pieces of material which can bend but not stretch and assuming the opening is on the top edge?
For a rectangular shape, this is commonly referred to as the paper bag problem:

For a rectangular shape, this is commonly referred to as the paper bag problem: