PE Chemical Practice Exam Questions

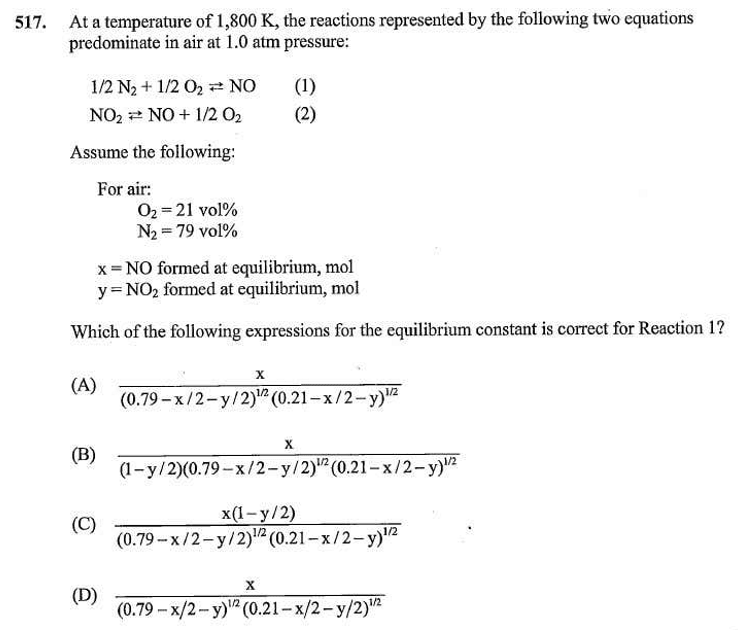
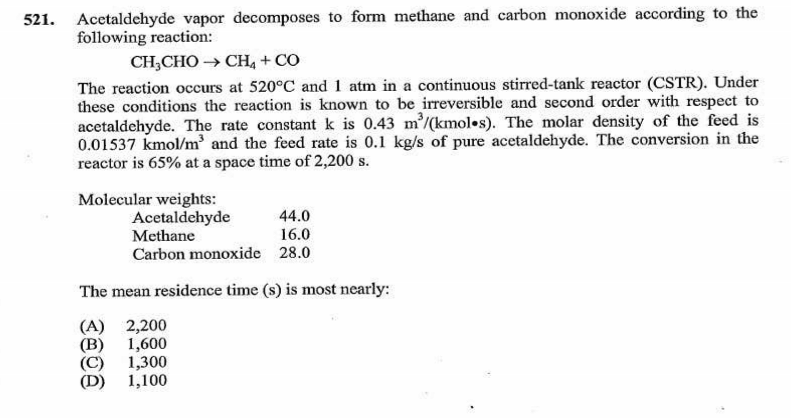
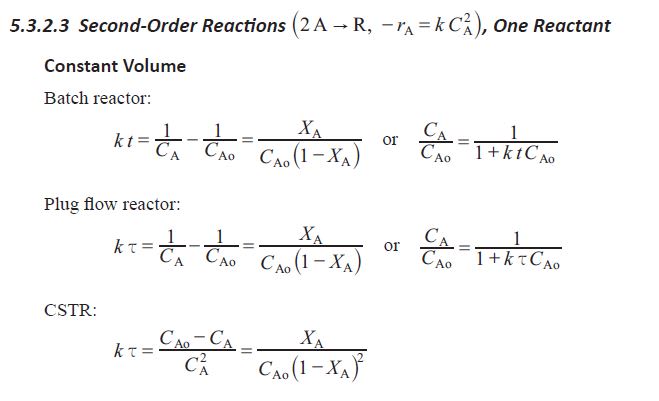
[AthlonXPme]

I am preparing for the PE Chemical practice exam and get stuck with these two questions. Please see the attached files. Does anyone have idea how to solve them ? Really appreciated if thought process can be shared.

 

[TiCl4]

I haven't worked the second problem, but here's the first:

Set up a normal equilibrium expression (all values in concentrations, but, this being a gas, vol% can substitute for concentration:

k = NO/(N2^0.5 * O2^0.5)

x=NO
y=NO2

The concentration of N2 is the amount of initial N2 minus consumption. For every mol of NO that is formed, 0.5 mol of N2 is used. Same for NO2.

0.79 - 0.5x - 0.5y = concentration of N2

Same for oxygen, but 1 mol of O2 is used for every mol of NO2 instead of 0.5 mol since each reaction uses 1/2O2.

0.21 - 0.5x - y

The concentration (vol%) of NO (x) is just the concentration, so it remains x.

So "A" would be my answer.

For the second question, if you do not have the PE reference manual, get it. It has spacetime equations for CSTRs, plug flow reactors, etc. That should be more of a plug-and-chug equation, but I don't have the formulae handy nor do I remember it that well. It may need simple integration since the decomp is 2nd order.

 

[AthlonXPme]

Thank you so much for such a detailed explanation. Now I understand the logic behind the 1st question. I should have written two separate equations: one for NO only and the other for NO2 via NO as the intermediate. This way helps better understand the molar balance to solve the problem.
Regarding the 2nd question, I do have the PE reference manual and this should be the formula for the 2nd order CSTR reactor. What confuses me is the mean residence time. Is it based on a certain conversion ? The question has already stated a space time of 2200 sec at the conversion of 65%. My understanding is space time is mean residence time by definition.

 

[TiCl4]

Spacetime is defined as reactor volume divided by inlet volumetric flow

The PE exam likes to throw a lot of extraneous information at you. Rate constant, molar density, etcs are all red herrings. The only relevant information is the decomposition equation and the spacetime.

For plug flow reactors and CSTRs, mean residence time equals spacetime if volumetric flow out = volumetric flow in.

Since this is a gas-phase reaction, and decomposition forms 2 mols of gas for every mol entrance, vout = 2 x vin. So mean residence time at 100% conversion would be 2200 / 2 = 1100. However, this particular CSTR has 65% conversion, so 1.65 mols out for every mol in. 2200 / 1.65 = 1,333 seconds, so "C" would be the answer.

 

[georgeverghese]

It isnt clear to me either what "mean" is here, since this is a 2nd order reaction, conversion is 65% of feed, and there is an increase in volume as reaction proceeds in the CSTR. It could be interpreted as either of:
a)Residence time based on volume midway through the reaction ie at 32.5% conversion
b)Residence time based on volume midway in time through the reaction. So if T is reaction time corresponding to 65% conversion, then use the volume at T/2.

Work out both and see.

 

[AthlonXPme]

At 65% conversion which means 0.65 mol CH3CHO is converted, should it lead to 0.65 x 2 = 1.3 mol out for every mol in ?
So if volumetric flow in not equals to volumetric flow out, how do we calculate the mean residence time ? I feel it should be somewhere between the number calculated based on the volumetric flow in and the number calculated based on the volumetric flow out.
Sorry. I am still very confused.

 

[TiCl4]

Georgeverghese,

This is a CSTR, not a plug flow reactor. Conversion at all points in the reactor is 65%.

OP, 0.65 mols of CH3CHO are converted, producing 1.3 mol of products. There are 0.35 mols remaining unconverted, for a total of 1.65 mols out. Vout = Vin * 1.65.

Again, one of the fundamental assumptions for a CSTR is that it is well mixed and the composition of the reactor is uniform. This means the outlet conditions are the same as reactor conditions, and that the mean residence time is calculated using Vout.

This isn’t quite correct, as composition gradients do exist in reactors, but for especially slow CSTRs with large spacetimes, it’s not a terrible assumption.

The PE exam only deals with the well-mixed assumption; otherwise all the given equations are not correct.

 

[georgeverghese]

@TiCl4,

Okay, got it, was working on batch reactor mode.

When you look at the wording of the question, it says space time at 65% conversion in this CSTR is 2200secs, so I would pick answer A !

 

[AthlonXPme]

@TiCl4,
Oh. Now I got it. Forgot to consider the unreacted CH3CHO in the outlet. It makes total sense that the mean residence time for CSTR should be calculated based on Vout. For PFR, I guess the mean residence time should be somewhere between the numbers calculated based on Vin and Vout.