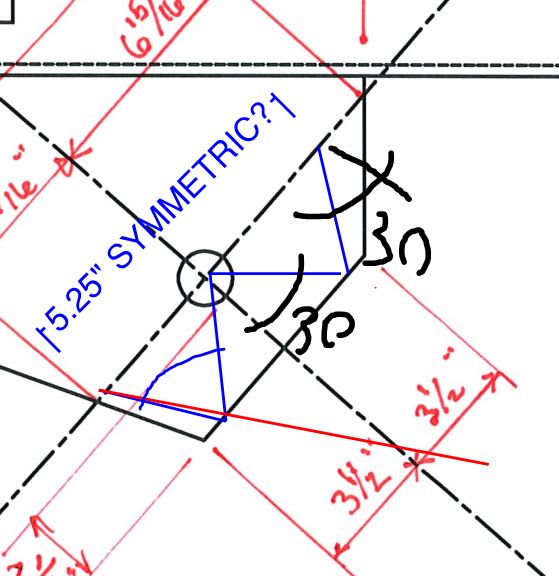
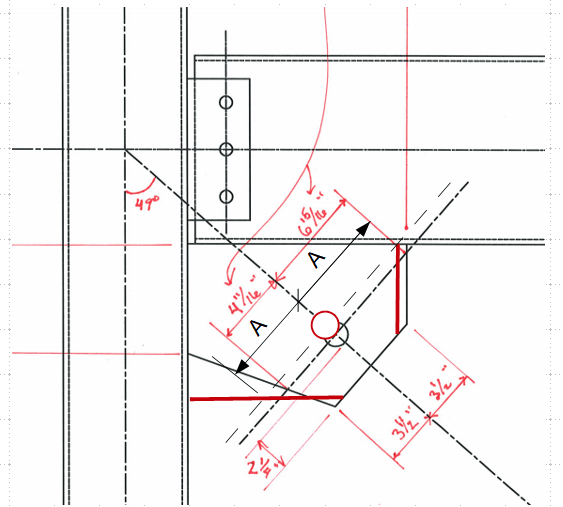
I am designing some braced frames which use rod x bracing. Where the tension rod meets the gusset plate, I plan on using a 3 1/2 clevis with a 1.75" Dia A36 pin. I can size the gusset plate geometry based on the uniform force method but I'm running into issues with the Pin connected member dimensional requirements. D5.2(a) says, "The pin hole shall be located midway between the edges of the member in the direction normal to the applied force." The attached sketch shows my dimensions as well as that code section. Do you guys have any suggestions for how to make these two requirements work together?
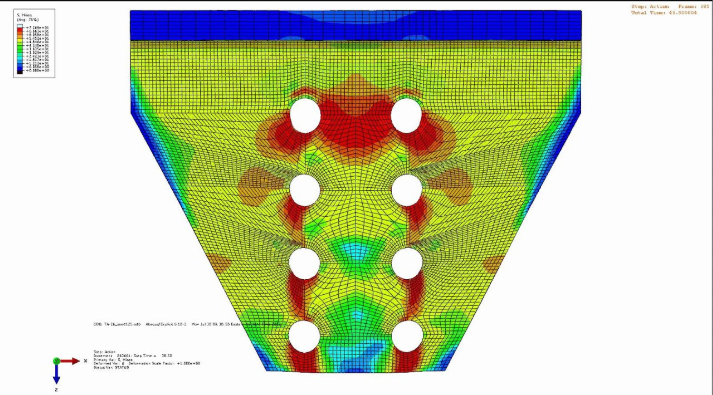
Also, in the AISC Example D.7 pin connected member design example, after doing their pin connection checks, they do a tensile yielding check on the full gusset width. In their example, the pin hole is centered in the gusset and they check tensile yielding across the full width of the plate. In our case would you agree with using our full 4 11/16" + 6 15/16" = 11.625" width for a yielding check? I would think of using a Whitmore Section but with only one hole I'm not sure how to go about that. The example problem is attached and pertinent areas clouded for reference.
Thanks.
Also, in the AISC Example D.7 pin connected member design example, after doing their pin connection checks, they do a tensile yielding check on the full gusset width. In their example, the pin hole is centered in the gusset and they check tensile yielding across the full width of the plate. In our case would you agree with using our full 4 11/16" + 6 15/16" = 11.625" width for a yielding check? I would think of using a Whitmore Section but with only one hole I'm not sure how to go about that. The example problem is attached and pertinent areas clouded for reference.
Thanks.