I'm working on a pressure vessel with a blind flange at its base, supported by a few large SHS's. To avoid FEA, can anyone direct me to a suitable hand-calc that could be used to assess the effect of the legs on the flange?
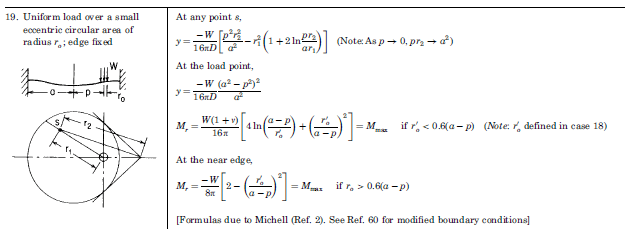
I'd been thinking of using Case 19 from Table 11.2 in Roarks 7th edition, to compare the deformation due to the reaction force from the legs to the magnitude of deformation from internal pressure, but despite it being for a fixed edge the results I'm getting have deformation at the perimeter - clearly something's not right there, and I'm struggling to find another resource.
I'd been thinking of using Case 19 from Table 11.2 in Roarks 7th edition, to compare the deformation due to the reaction force from the legs to the magnitude of deformation from internal pressure, but despite it being for a fixed edge the results I'm getting have deformation at the perimeter - clearly something's not right there, and I'm struggling to find another resource.