eli28
Aerospace
- Oct 20, 2019
- 109
hello,
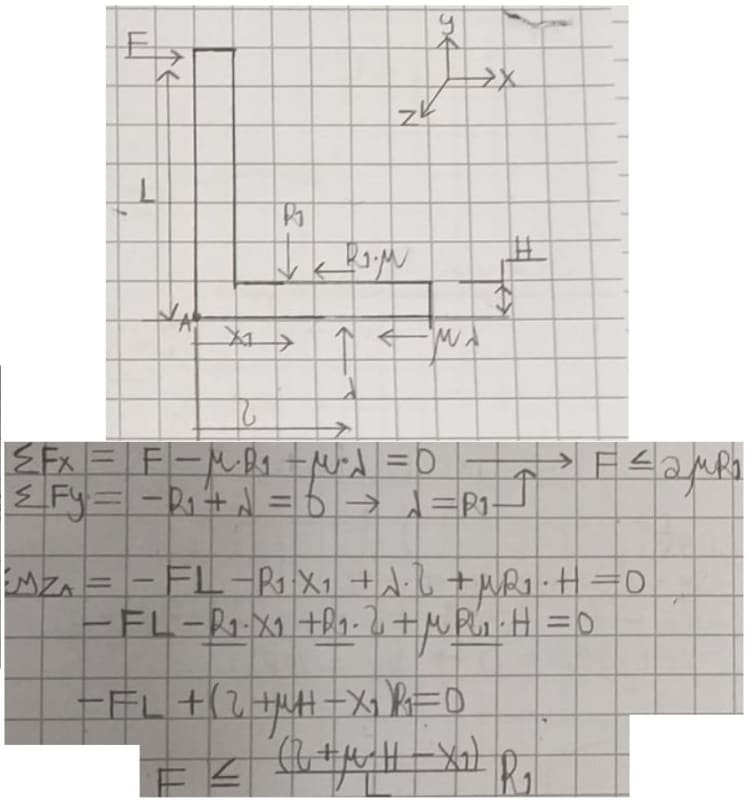
I am trying to figure out what's wrong with a free body diagram I wrote down.
I added a pdf file with a description of the problem.
please give your advice
Thanks
I am trying to figure out what's wrong with a free body diagram I wrote down.
I added a pdf file with a description of the problem.
please give your advice
Thanks





![[dazed] [dazed] [dazed]](/data/assets/smilies/dazed.gif) .
.
![[sad] [sad] [sad]](/data/assets/smilies/sad.gif) and not sure about the right solution since there were some different answers.
and not sure about the right solution since there were some different answers.