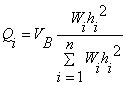Seismic Force Distribution 3

[Bh.Burhan]

Hello all,

Does the seismic force distribution change between the North-South direction and the East-West direction? If I have the same seismic resisting force system for the both directions in my building. The building is rectangular moment resisting frame without irregularities.

Thanks,

 

[r13]

As base shear, V[sub]B[/sub], is constant regardless of direction, do you see any directional reference from equation below:

Where Qi = Design lateral force at floor i
Wi = seismic weight of the floor i
hi = height of the floor i from the base
n = number of storeys of the building at which masses are located.

 

[Bh.Burhan]

So the force distribution along each side of the building diaphragms will not change.

Thank very much!

 

[Rabbit12]

That's not necessarily true. The seismic force is the same in each direction if you have the same SFRS but if could be applied in a different location if you have a rigid diaphragm.

 

[Blackstar123]

How come you have same base shear in both directions if your building is rectangular in plan?

Fundamental period should be different in both direction unless code specified maximum period is governing.

 

[JAE]

The base shear (for equivalent static procedure) is the same in both directions.

The vertical distribution of the forces, at each level, usually are the same as the mass of each level and the height of each level is the same per the distribution formula that retired13 presented.

For some buildings, however, the building could have unique features that affect the flow (response) of the structure in different directions. Variability of stiffness at various levels, layout, number and relative stiffness of moment frames could be different, etc.

So the base demand in each direction, given the same R value in each, can be the same, but the response and load path on the resistance side might be very different.

 

[Rabbit12]

Agree JAE.

Blackstar, the Cs value is usually independent of T unless you hit the maximum. I usually approximate the period per the prescriptive requirements in the code. So if you have the same system in each direction your maximum would be the same too.

 

[Blackstar123]

the Cs value is usually independent of T unless you hit the maximum.

My understanding is completely opposite to this statement. Cs value is dependent on the period of the structure in the direction under consideration.
Period of the structure is the factor that determines the structure response lies in which part of the response spectrum and consecutively, what should be seismic response coefficients the structure will experience and should be designed for.

 

[Rabbit12]

Blackstar,

Cs=S[sub]DS[/sub]/(R/Ie) unless that value exceeds the maximum which is tied to the period of the structure. Rarely have I used the actually period of a building to determine the maximum but it could be done which could change the distribution in orthogonal directions.

V=Cs*W

So generally using the ELF method your base shear doesn't change based on T.

 

[JoshPlumSE]

Blackstar -

I think of it the same way as you do. But, that's just because the 1997 UBC had the equations switched. The base equation was the equation with Cv and T, and the max equation was the one based on Ca.

With ASCE 7, however, the base equation is the one based on Sda, and the Max equation is the one based on Sd1 and T.

Tomato, to-mah-to.....

 

[Rabbit12]

JoshPlum, why do you say tomato, to-mah-to? Seems the way we calculate seismic force has changed. I can't imagine may jurisdictions are still using the 1997 UBC.

 

[JoshPlumSE]

Rabbit12 -

Actually the two sets of equations are essentially identical. The 1997 UBC seismic code was based off of the NEHRP equations at the time, they just re-jiggered some of the equations a little (as I understand it). Then the IBC and ASCE used the NEHRP equations exactly as they were.

Acceleration based equation:
Cs = Sda*I/R (ASCE/IBC)
Cs = 2.5*Cv*I/R (97UBC)

So, these are the same equations if Sda = 2.5 Cv

Velocity based equation:
Cs = Sd1*I/RT (ASCE/ IBC)
Cs = Cv*I/RT (97UBC)

So, the equations are the same if Sd1 = Cv.


Same basic seismic theory. Just changed the variables a little. Now, the long period equation (displacement based equations on T_L) doesn't exist in the UBC. So, that's a big change, but very few buildings are controlled by the displacement based equations.

 

[r13]

Blackstar and JoshPlum have a valid point, the key is how the fundamental period is determined.

Using approximate method,
Ta = Ct*h[sub]n[/sub][sup]x[/sup], in which Ct & x = constants for building framing type
Vertical distribution factor,
Cvx = Wx*hx[sup]k[/sup]/ΣWi*hi[sup]k[/sup], in which k = a constant depends on the magnitude of the fundamental period, Ta
Finally, seismic force in each level,
Fx = Cvx*V[sub]B[/sub]

Note in the procedure above, everything is non-directional. However, you may chose to calculate/derive fundamental period for each direction according to the geometry, or stiffness, differences. Which would be more realistic, I think.

 

[Blackstar123]

Well explained, JoshPlumSE.

 

[Rabbit12]

Josh, help me understand what S[sub]da[/sub] is. There is no such term in ASCE 7-16 that I see for calculating seismic force per the ELF procedure.

I'm trying to understand how the seismic force distribution using the ELF in the current ASCE7 is dependent on the period of the structure, except for the maximum C[sub]s[/sub] value in ASCE7 12.8.1.1. Assuming the OP used the approximate period of ASCE7 12.8.2.1 the period would be identical in each direction; same system same height. Using the equation referenced by retired13 above would give the OP the same lateral force in each direction.

 

[spieng89]

Seismic load distribution, as in percentage of load distribution, should be the same in either direction, but base shear doesn't have to be if you have different seismic force resisting system in the different directions (different R values). ASCE allows you to consider different R values in different directions.

 

[r13]

Rabbit12,

I think S[sub]da[/sub] is a typo of S[sub]ds[/sub].

For directional concern, it is interesting to find the approximate period equation below that clearly indicates the influence of building dimension in direction of motion.

Ta = 0.09*h/sqrt(d), h = building height (m); d = building length in direction of acceleration (m)

Ref. - International Research Journal of Engineering and Technology (IRJET), 2017

 

[JAE]

spieng89 - you are correct on that point!

 

[JoshPlumSE]

Rabbit -

Retired13 is correct. Sds is the short period acceleration term for ASCE. I was going off of memory (as I don't have any of my references with me right now), and I used Sda by mistake. That's because I was thinking "acceleration based" behavior (which is why the UBC called the similar variable Ca).

For what it's worth, this always makes more sense to me when I look at the tripartite plot of the ASCE / IBC equations. Which I'll post in a follow up post (from my other computer).

 

[JoshPlumSE]

In the image below you can see how the Sds equation represents a region of constant acceleration in the design spectra. The Sd1 equation represents a region of constant velocity, and the TL equation represents a region of constant displacement.

I like this type of plot because it helps to understand the theory behind why the seismic equations are the way they are.