Settlement vs Foundation Size

[MramJ]

Hey everyone,

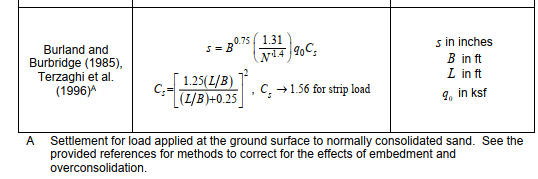
In the CFEM, there is an equation by Burland and Burbidge (1985) for foundations on normally consolidated coarse-grained soils:
\[ S = B^{0.75} \times \frac{1.6}{N_{60}^{1.4} \times q} \]

I believe that this equation is based on observed data, but there are a couple of things I'm not sure I understand:

1. The equation seems to suggest that as the foundation width \( B \) increases, the settlement \( S \) also increases. I understand that a larger stress bulb covers a larger volume of soil with a larger footing, but isn't the stress lower, assuming the same load on both smaller and larger footings? Isn't this similar to having wider tracks on heavy machinery to distribute loads and reduce settlement?

2. Taking the equation to an extreme, if we have a very small foundation width (close to zero) and a massive load, the equation suggests almost zero settlement. This seems unlikely in a real-world context.

I'm curious if there are specific limitations or conditions under which this equation should be applied that I might be missing. Maybe there's a range of values for \( B \) where this equation is most relevant?

Thank you

 

[geotechguy1]

>1. The equation seems to suggest that as the foundation width \( B \) increases, the settlement \( S \) also increases. I understand that a larger stress bulb covers a larger volume of soil with a larger footing, but isn't the stress lower, assuming the same load on both smaller and larger footings? Isn't this similar to having wider tracks on heavy machinery to distribute loads and reduce settlement?

Little q is a unit load in kpa not the load in kN, so if you increase B and apply the same q (which would result in a larger Q) then yes, settlement would increase. However, if you apply the same Q (reuslting in a smaller q) then a bigger footing would reduce settlement.

 

[MTNClimber]

If it’s not apparent, Geotechguy’s answer applies to your second question. The smaller the footing while maintaining the same bearing pressure indicates a smaller force (load) on the footing. This means there will be less settlement.

 

[CarlB]

You are right to question implications of the equation. What the other responders failed to note, if bearing pressure q is increased, other variables unchanged, the settlement would decrease. This is obviously wrong.

In a review of other references to the method, the equation you posted is incorrect.
The standard form I find is that q is in the numerator vs the denominator, the coefficient is 1.706 vs 1.6, and the exponent is 0.7 vs 0.75.

See eqn #15 in their paper:

https://www.researchgate.net/profil...tlement-of-foundations-on-sand-and-gravel.pdf

 

[MTNClimber]

CarlB: you’re correct. I’m unfamiliar with this method, but I should have noticed that. Either way, the theory is still the same.

 

[geotechguy1]

I agree with CarlB, it looks like an error in the CFEM perhaps?

In Eqn 4 in the reference paper q' is in the numerator.

 

[MramJ]

Thank everyone, and sorry for wasting your time on this. I am not really a Geotechnical engineer, but work with few and I am trying to understand how things work in the geotech world. I agree with CarlB, I believe there is a typo in this equation, but I don't access to the CFEM to confirm, as I was given this by a junior engineer who also mentioned that settlement will always increase as the size of the footing increase, which I found counter intuitive when you have a constant load that can be distributed over a larger footing, assuming constant soil layer. So I am sorry again about this, I should check my sources before asking.

 

[MTNClimber]

I just came across this in UFC 3-220-10 (DM7.1):