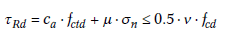VT17
Structural
- Apr 27, 2018
- 14
Hello All. I've read through several different posts regarding shear friction and I haven't found where this particular topic has been asked regarding shear friction. The closest thread I found was this one: Link.
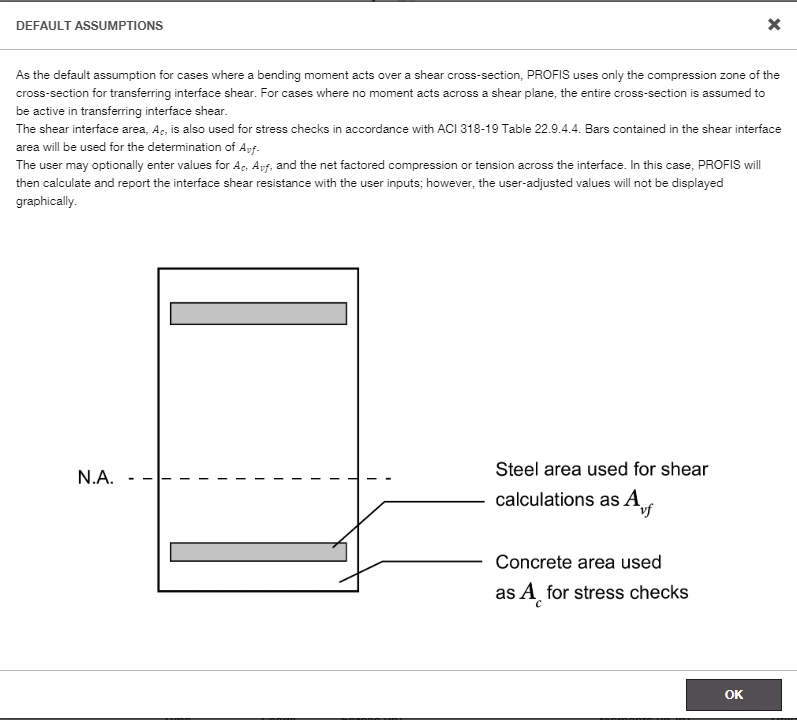
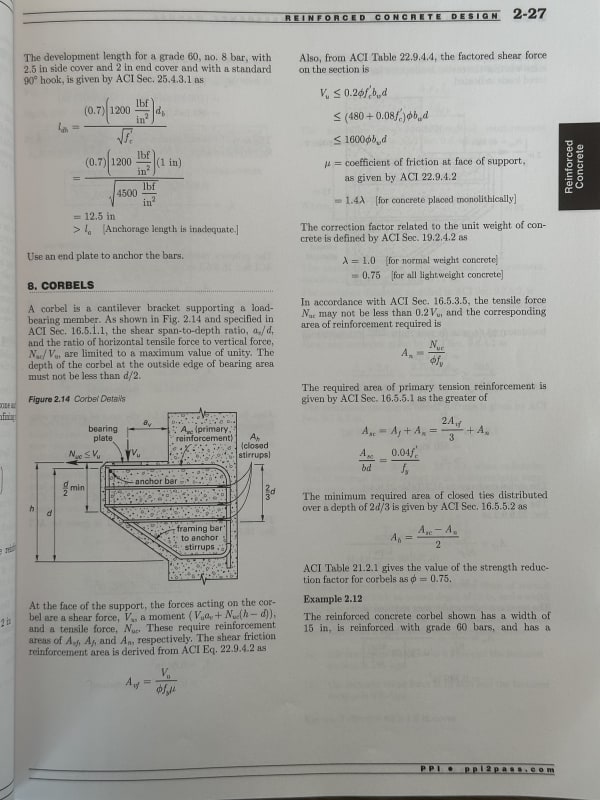
The question: What is Ac when determining the maximum Vn across the assumed shear plane per Table 22.9.4.4 (ACI 318-19) when a moment acts on a shear plane?
My thoughts: The definition of Ac per chapter 2 is the area of concrete section resisting shear transfer. The name of the above referenced table further makes me think that Ac is the area of the entire shear plane. In other words, if you have a section with a width of "b" and a height of "h", then Ac = b x h. However, if a moment acts on the shear plane where one side of the neutral axis will be in tension, should the depth of the section that contributes to Ac be reduced from "h" to the depth of the compression block "a" so that Ac = b x a? Or perhaps this is being too conservative and "h" should be reduced to "d", the distance from the extreme compression fiber to the the centroid of the longitudinal tension reinforcement?
Thank you in advance for reading.
The question: What is Ac when determining the maximum Vn across the assumed shear plane per Table 22.9.4.4 (ACI 318-19) when a moment acts on a shear plane?
My thoughts: The definition of Ac per chapter 2 is the area of concrete section resisting shear transfer. The name of the above referenced table further makes me think that Ac is the area of the entire shear plane. In other words, if you have a section with a width of "b" and a height of "h", then Ac = b x h. However, if a moment acts on the shear plane where one side of the neutral axis will be in tension, should the depth of the section that contributes to Ac be reduced from "h" to the depth of the compression block "a" so that Ac = b x a? Or perhaps this is being too conservative and "h" should be reduced to "d", the distance from the extreme compression fiber to the the centroid of the longitudinal tension reinforcement?
Thank you in advance for reading.