swearingen
Civil/Environmental
- Feb 15, 2006
- 667
I'm quite sure I'm pressing the wrong button on this thing, but I can't seem to find the button...
My situation:
- Trussed industrial tower with various types and sizes of spread footings underneath.
- Lateral wind and seismic applied.
- Structure consists of steel members.
- Footings consist of concrete solids.
- Springs using a subgrade modulus of 100 pci for the stiffness (100pci x 144in^/sqft x 12in/ft / 1000lb/k = 172.8 k/ft spring stiffness per node associated with 1 square foot).
- Compression only spring specification added to the nodes at bottom.
- Lateral restraint at top of pedestals applied (grade beams between columns).
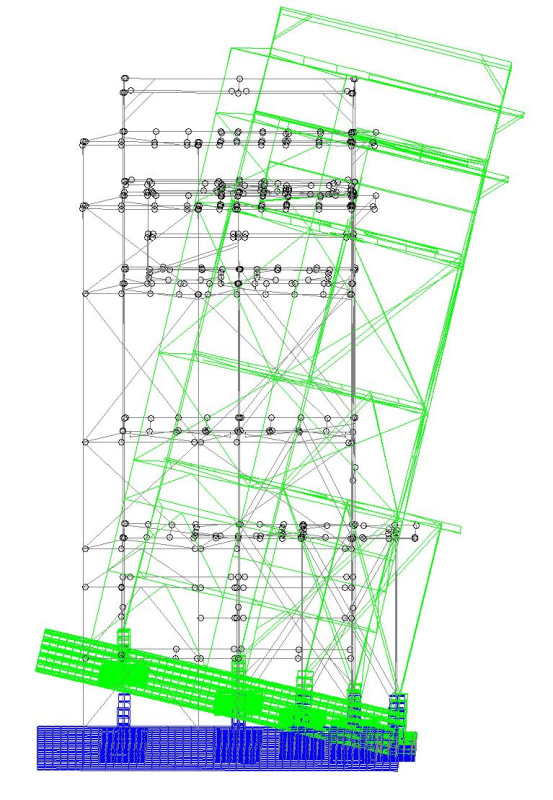
I'm getting a deflected shape that shows the entire tower rising off the ground by hundreds of inches. Things I've checked:
- Selfweight is vertical axis Y and -1.
- All loads have correct signs; gravity and equipment loads are all -Y.
It's obviously not right (as you can see from the picture). Where else can I look for errors?
Thanks in advance,
Frustrated Engineer
-5^2 = -25 ;-)
My situation:
- Trussed industrial tower with various types and sizes of spread footings underneath.
- Lateral wind and seismic applied.
- Structure consists of steel members.
- Footings consist of concrete solids.
- Springs using a subgrade modulus of 100 pci for the stiffness (100pci x 144in^/sqft x 12in/ft / 1000lb/k = 172.8 k/ft spring stiffness per node associated with 1 square foot).
- Compression only spring specification added to the nodes at bottom.
- Lateral restraint at top of pedestals applied (grade beams between columns).
I'm getting a deflected shape that shows the entire tower rising off the ground by hundreds of inches. Things I've checked:
- Selfweight is vertical axis Y and -1.
- All loads have correct signs; gravity and equipment loads are all -Y.
It's obviously not right (as you can see from the picture). Where else can I look for errors?
Thanks in advance,
Frustrated Engineer

-5^2 = -25 ;-)