Stability Analysis for Walls/frames that support cathedral / vaulted / scissor trusses 3

[StrEng007]

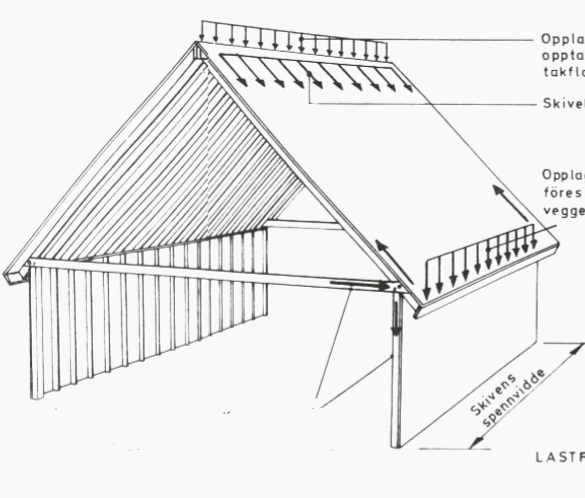
I’ve seen a lot of discussion about scissor/vaulted trusses here and there seems to be a consensus in the overall design approach.

1. Try to restrict the truss from moving (due to truss deflection) and impart a large thrust load on the supporting structure.

2. Allow the truss to displace as a pin-roller connection and check to make sure your walls can handle the displacement.

It looks like most of you have agreed that making a structure stiff enough to withstand the required truss deflection would be a futile exercise. Also it’s not so much the type of truss connection that allows this displacement (ie using one of those Simpson “slip connections”) but rather the lack of infinite stiffness from a wall support that give you the theoretical roller (makes more sense to me with wood than the scenario I'm about to present).

In both situations, the stiffer the truss members and the less overall deflection the truss experiences, the less the horizontal reaction if the truss was pin-pin, and the less the horizontal deflection for a pin-roller.

Good so far? Is there anything I’ve misinterpreted?

Most of my projects don't utilize wood construction for the wall system. 99% of the time I have bond beam/tie beam with CMU or concrete construction. Sometimes I even have concrete portal frames as discussed below. The way I treat most of my regular truss type conditions/non-scissor type (that is, in order to stay in line with the truss designer utilizing a pin-roller approach) is to consider that most of Simpson's truss connectors will deflect in the F1 direction, no matter what the given F1 load is. Here is some language from the Simpson Catalog:

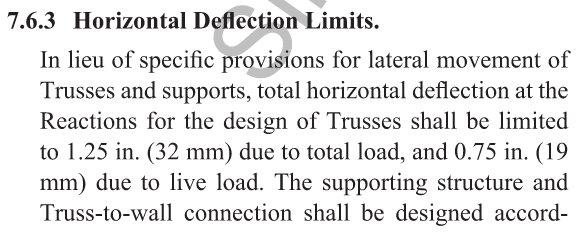
So given the fact that a typical connector has up to an 1/8" deflection and the Truss Design Documents for typical trusses have a horizontal creep total (CT) that doesn't exceed these values, you can assume the designated end of the truss is able to displace. [highlight #FCE94F]You usually don't find engineers who are arguing that their tie-beams are laterally displacing enough to provide a roller support.[/highlight]. I realize this invalidates what some of you had said about a truss not being able to displace due to friction at the connection, or the diaphragm working in unison. To be honest, I don't know how to qualify the fact that all our truss designers use pin-roller if not for this.


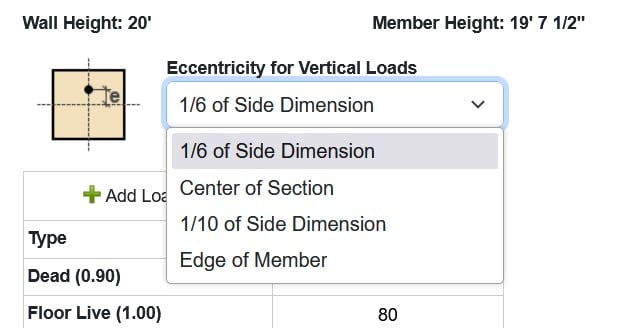
So how do we handle the stability analysis in a situation like this? To better explain my situation, I’m looking to do a cathedral style roof that sits on a concrete portal frame with storefront infill below the frame. So I will have lateral loading in the plane of the portal frame (F1), gravity load from the roof truss, and an assumed F2 due to wind load on the walls (windward, leeward, side wall) in addition to a F2 from the thrust load of the truss (btw its' so interesting how all this is going on but rarely gets illustrated). With a cathedral ceiling, I don't see how I can take the same liberties that I do for typical a common truss. So how should I handle the stability analysis?

Also:
When doing cathedral style roofs:
1. Is is common for the architect or GC to put the truss designer and structural EOR in connection prior to the development of construction documents. So often I'm supposed to wait a month out until the truss design can get me the TDD reactions, and for a truss like this it's putting the cart in front of the horse.
2. Is there a better way to handle the trust reactions? I must have no tension rods, no ceiling at the tie-beam elevation, a maximum sloped vault inside the structure, no ridge beam or columns to break up the spans, and there cannot be buttresses or pier cast into the wall (ie, inside face of walls all have to be uniform).

 

[lexpatrie]

I'm just having one of those "need more pictures" caveman moments. Wish I could contribute.

I believe the TPI for lateral deflection is 3/4" and you can't realistically do much with the truss to reduce that horizontal deflection. It's the nature of the structural system that it spreads. Same as rafters with an elevated tension tie (NOT a collar tie! Collar ties are in the top third of a roof, tension ties or elevated ceiling joists are in the bottom third).

You also can't realistically do much to prevent that outward thrust because the wall just doesn't do that, it doesn't have the rigidity (at the base, as well as in the wall), and you also typically can't get the necessary horizontal strength in the connection anyway, so the wall deflects. You could conceivably check the wall given a 3/4" deflection at the top as an eccentric load, and with masonry, that may not have much affect on the design. Put in a slip connector for uplift.

Regards,
Brian

Side note - if this is a prefab wood truss, the SBCA is where you'd look for a technical discussion, or, your friendly neighborhood forensic (structural or at least civil) engineer who specializes in wood design and wood construction defect. Ideally one who actually took a class in their education on wood design, and there aren't that many of us around.

 

[RontheRedneck]

This subject is brought up with me from time to time.

I'd like to start out by saying that in the 35+ years I've been designing trusses, I have never been called to look at a job that had problems that had been caused by scissor truss deflection.

Someone mentioned the TPI limit - It's actually 1.25".

I assume most of you already know what can be done to reduce deflection if you want to - Increase the TC pitch, decrease BC pitch, and increase chord sized and/or grades.

But the customer has to be willing to go along with that. And they often aren't.

If you start bumping lumber sizes/grades someone has to be willing to pay for it. That's often a point of contention.


I'm a fan of the Simpson scissor truss clips that Lexpatrie posted a picture of. I believe the theory is that you set the trusses, but leave the nails loose in the slots. Put the roof on, so most of the deal load is on the trusses. Then re-plumb the walls and drive the nails tight.

After that you'll still have live load deflection to deal with. But the dead load has mostly been taken care of.

 

[WestLevel]

Arches thrust. Trusses dont.

 

[XR250]

I'm a fan of the Simpson scissor truss clips that Lexpatrie posted a picture of. I believe the theory is that you set the trusses, but leave the nails loose in the slots. Put the roof on, so most of the deal load is on the trusses. Then re-plumb the walls and drive the nails tight.

I think expecting the contractor to leave the nails loose and then to go back and drive the nails is a fools errand. That is why I never specify these.

 

[WestLevel]

Do you guys design the bearings of truss's on truss plates to resist the end rotation of the truss load?

 

[RontheRedneck]

WestLevel, I'm not sure if your question was directed at me or not. If it was, I have no idea what you're asking.

 

[Eng16080]

In OPs case, that clip doesn’t make much sense considering that the truss is being relied on to resist wind forces pushing into the wall.

If the wall was not relying on the roof for lateral support, perhaps then it might be of some use, but I find it unlikely that this detail gets constructed correctly. Even if the builder installs the clips correctly without the nails fully hammered down and positioned correctly within the track, the interior and exterior finishes would need to be constructed in a manner which allows the horizontal movement. Besides that, there will be some friction force between the truss and wall top plate which might also prevent the detail from working as intended.

 

[lexpatrie]

I'm going to say that the Simpson TC24 the nails aren't driven flush. At any point. They'll perhaps squeak. I believe they are tested in the "loose" condition. I believe it's rated for uplift and load perpendicular, so as a mild diaphragm force transfer.

Interesting point about the wind on the wall, though. Ron can you give us an excerpt of the 1.25" horizontal spread from the TPI?

 

[bones206]

You could conceivably check the wall given a 3/4" deflection at the top as an eccentric load, and with masonry, that may not have much affect on the design.

FWIW, I think it is a common practice for wood stud walls to be designed including a D/6 bearing eccentricity. It might make sense to bump that up accordingly when you have a roof system with significant lateral spread under load.

 

[RontheRedneck]

Lexpatrie said: "Ron can you give us an excerpt of the 1.25" horizontal spread from the TPI?"

No idea what you're looking for.

 

[bones206]

From the 2014 version:

 

[lexpatrie]

That D/6 is done by some folks, I wouldn't call it standard practice or code, it's an optional check box in Forte as I recall. I may be out of date, though.

It think quite a bit of the Weyerhaeuser load tables include that effect, however. It makes more sense to me as something for isolated deck posts.

On this particular project, it makes some physical sense, and you'll have to get some stabilization of the top of the wall when the nails go into bearing against the slots to resolve that wind force on the wall.

Are we in a snow region? And then lemme ask "live load" meaning floor live load, rather than roof live load?

 

[bones206]

I have only been practicing with wood design for 3-4 years now, so I'm not an expert by any means. But I have seen that D/6 eccentricity considered pretty often in wall stud designs. It is a default setting in Woodworks Sizer.

Regarding live load in the deflection provision, the TPI 1 document defines live, rain and snow under the umbrella label of live load.

 

[XR250]

Never used D/6 - never will

 

[lexpatrie]

I think it's possibly coming, in terms of code, but when it comes to a wall stud, there's ... anyway. There's so many of them when it's wall studs, that seems unduly punitive.

It makes more sense to me on posts.

Sizer is Canadian in origin, so it's perhaps part of the Canadian code. I wouldn't know.

 

[wth]

Don't do much wooden structures, but could you, in theory, utilize the roof as a semirigid slab to transfer this horizontal force to the gable walls and limit the deflection?

 

[XR250]

Here is one I looked at yesterday. Been there 12 years with no signs of any distress. The beams are perfectly straight. It is about 22 ft x 22 ft. So, yea, the roof does act as a semi-rigid slab.

 

[lexpatrie]

There's something going on at least potentially, though a gable and a hip roof aren't all that comparable. I've thought about it, since I had a building official flag something like this in the real world, but there's no obvious "out" for the end reaction v = wL/2 to hold it up. I suppose the rafter to top plate connection is doing it, one rafter at a time, .... the one I have very deeply does not work in traditional static load paths and has stood for 100 years. There's construction that hasn't fallen down yet and what we can prove via structural analysis and the two don't 100% overlap. That idea of using the sheathing as a sort of deep plywood beam comes up a lot, particularly from "outside" the structural engineering community.

Actually, I have two projects like this, both 100 years old or more. (i.e. they are wood planks, not plywood/OSB and not blocked)

 

[JAE]

The sheathing-as-a-deep-plywood-beam exists.
The problem is, we engineers don't typically have the tools, or the time, to include this effect in our calculations - we like discreet load paths and this ain't one of them.

 

[wth]

Fair point JAE, even detailing a couple of simple wood shear walls could blows our budget, a tall roof beam would in principle be more of the same.