CorporalToe
Civil/Environmental
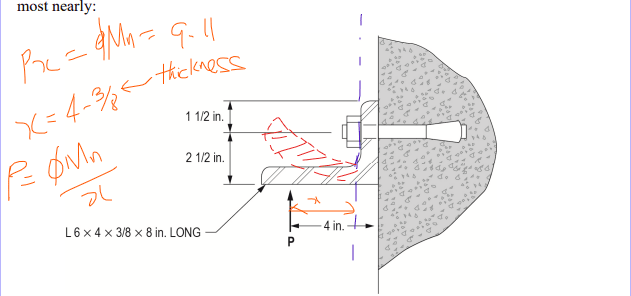
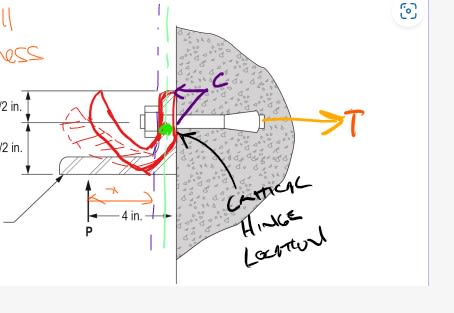
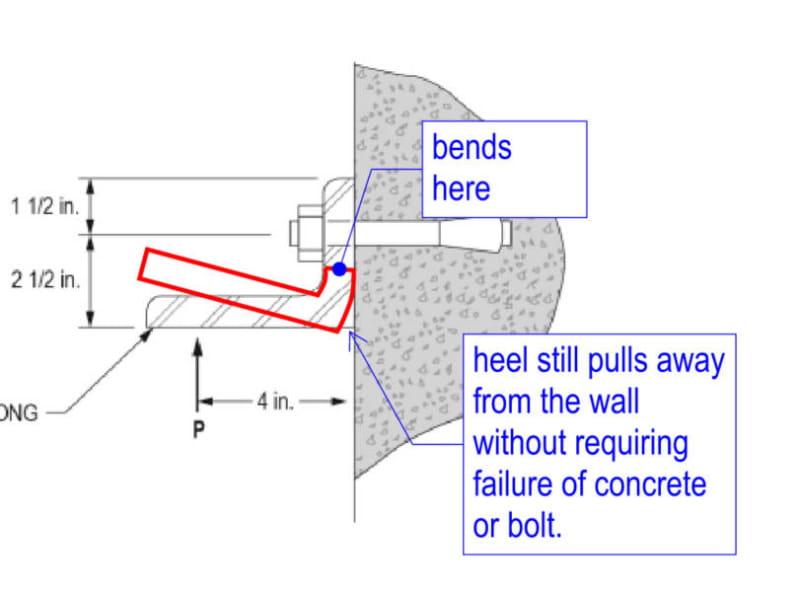
Hello I am studying for my PE Civil Structural Exam. I was wondering for this question why would you not use the yielding formula from
AISC 360-16 F.10:
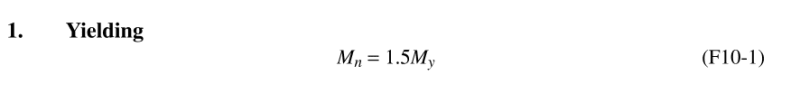
Question:
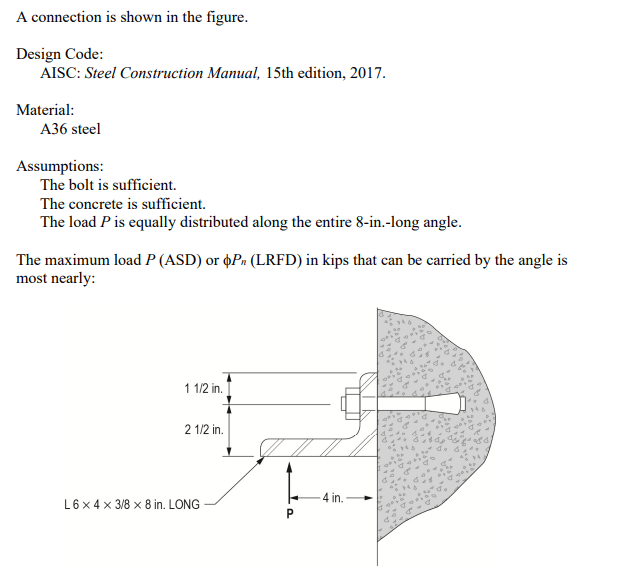
Solution:
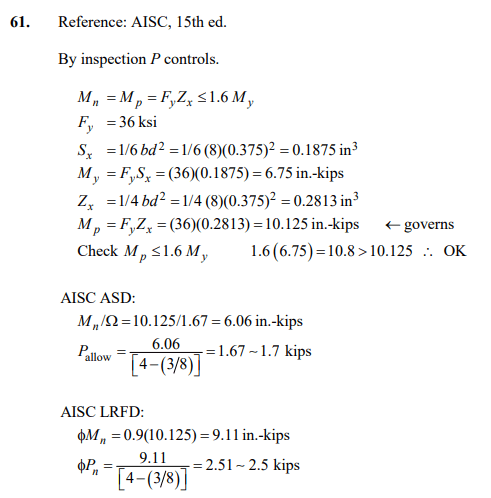
AISC 360-16 F.10:
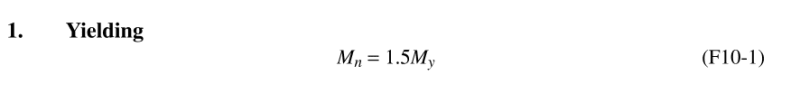
Question:

Solution: