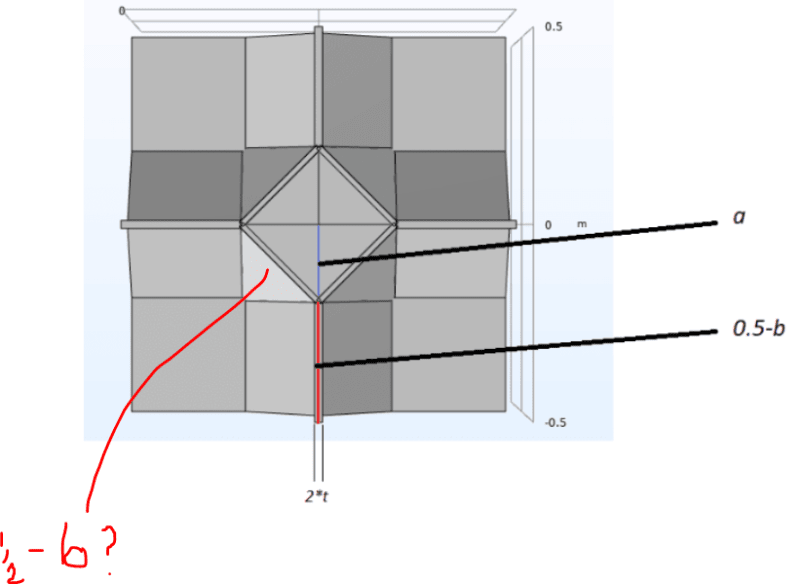
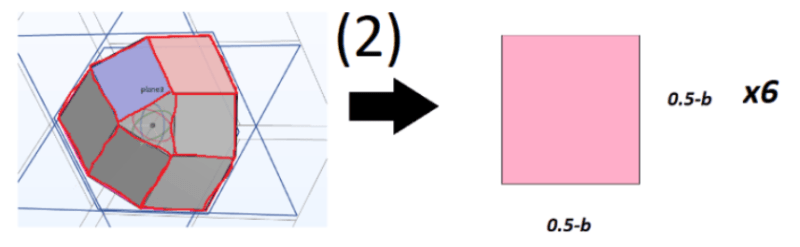
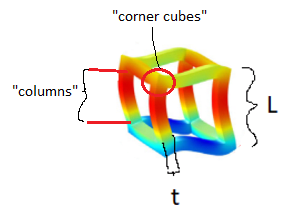
I am trying to find the relation between geometric parameter "a" and "b" using that the volume fraction is 0.2.
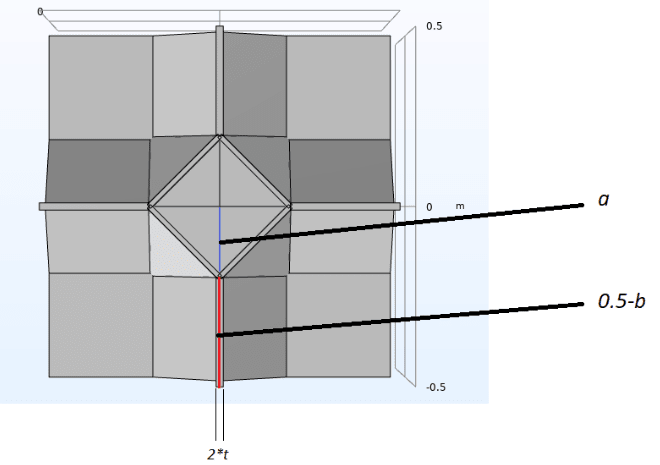
Fig 1: This is the geometry I am working with, its symmetric about xyz axis so I divide it into 8 similar parts:
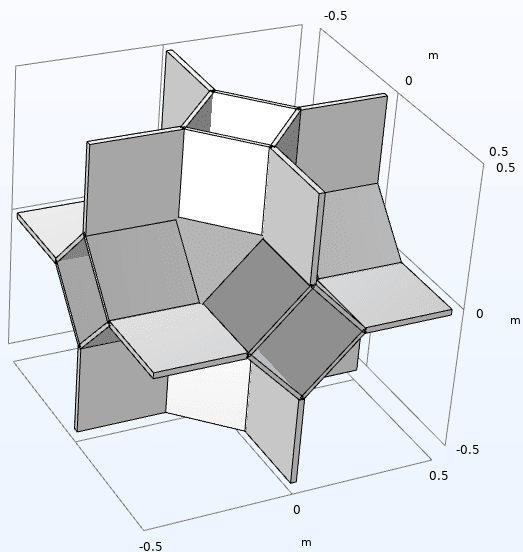
Fig 2: Looking at 1/8th of the geometry - I want to find the volume of this part which consists of:
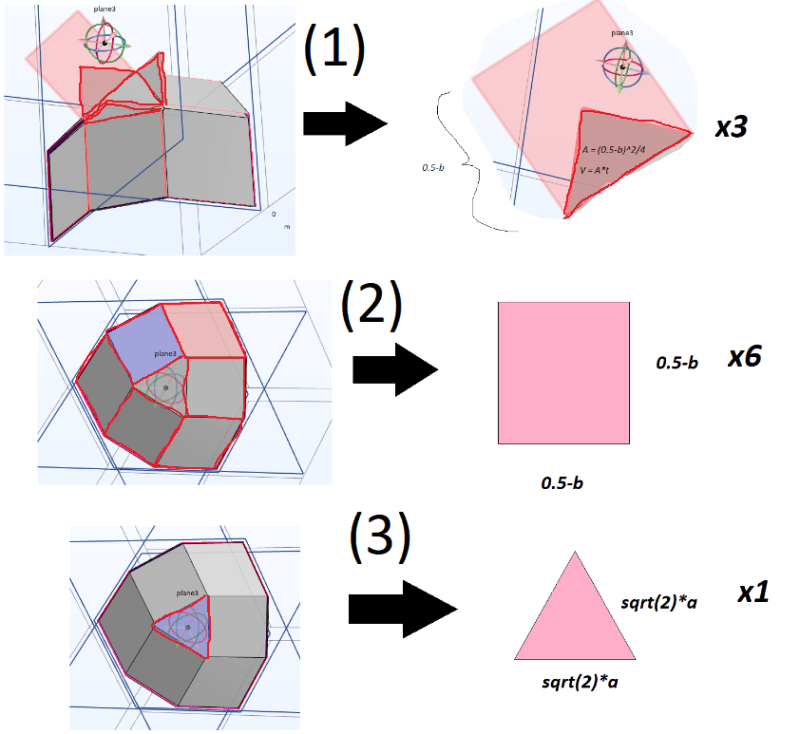
x3 triangles with thickness t (1),
x6 rectangles with either thickness t or t/2 (2)
x1 triangle with thickness t (3)
I calculate the volume of these parts, add them together and it comes out to be:
5.25*t*(0.5-b)^2 + sqrt(3)*a^2*t/2
The volume of the 1/8th part would be (a+0.5-b)^3 if it was a regular cube:
So the volume fraction would be given as:
[5.25*t*(0.5-b)^2 + sqrt(3)*a^2*t/2]/[(a+0.5-b)^3] = 0.2
My problem with this is that I get 3 parameters; "a", "b" and "t". If I only had "a" and "b" in the equation without "t" for example I could easily find the relation between these two parameters and maybe ended up with something like 0.78*a = b.
Does anyone have suggestions on how I should go about doing this, I am kind of stuck here right now.
Fig 1: This is the geometry I am working with, its symmetric about xyz axis so I divide it into 8 similar parts:

Fig 2: Looking at 1/8th of the geometry - I want to find the volume of this part which consists of:

x3 triangles with thickness t (1),
x6 rectangles with either thickness t or t/2 (2)
x1 triangle with thickness t (3)
I calculate the volume of these parts, add them together and it comes out to be:
5.25*t*(0.5-b)^2 + sqrt(3)*a^2*t/2
The volume of the 1/8th part would be (a+0.5-b)^3 if it was a regular cube:

So the volume fraction would be given as:
[5.25*t*(0.5-b)^2 + sqrt(3)*a^2*t/2]/[(a+0.5-b)^3] = 0.2
My problem with this is that I get 3 parameters; "a", "b" and "t". If I only had "a" and "b" in the equation without "t" for example I could easily find the relation between these two parameters and maybe ended up with something like 0.78*a = b.
Does anyone have suggestions on how I should go about doing this, I am kind of stuck here right now.