EdwardNigma
Aerospace
- Oct 18, 2023
- 18
Hello guys. I know this has been asked many times but I’m interested in knowing the main differences between von Mises, Tresca, and Principal Stress theory and when to apply them.
The reason why I ask is because I've mostly worked in aerospace and I first started my career out of college as a stress engineer at a small company. I used extensive FEA to perform static and dynamic analysis and applied von Mises stress criterion to calculate MS against Ftu material allowables (metallic structures steel, aluminum and Inconel). Then I worked performing stress analysis of airframe fuselage metallic (aluminum) repairs for an OEM and used max principal stresses to compare against Ftu in MMPDS to calculate margins of safety. General guidelines suggest using von Mises for ductile materials, not max principal stresses, but I used principal stresses in this case.
After some time, I spent the majority of my career as a designer and leading up to this I didn't question the senior stress engineers in my short stint as a stress engineer. When I returned to stress analysis, I worked on nacelle repairs and, in one occasion, I had to calculate the margin of a blended metallic fitting, which was certified using FEM in the SCN using von Mises stress criterion. Due to limited data, my approver said it would be sufficient to compare the change in area and apply a knockdown factor to the FEM data and refer to Fty (yield), not Ftu (ultimate), due to the use von Mises stress during certification. That made me become curious about different failure modes but whenever I questioned things with other engineers I only seem to get in trouble as I discredited them from doing things the right way.
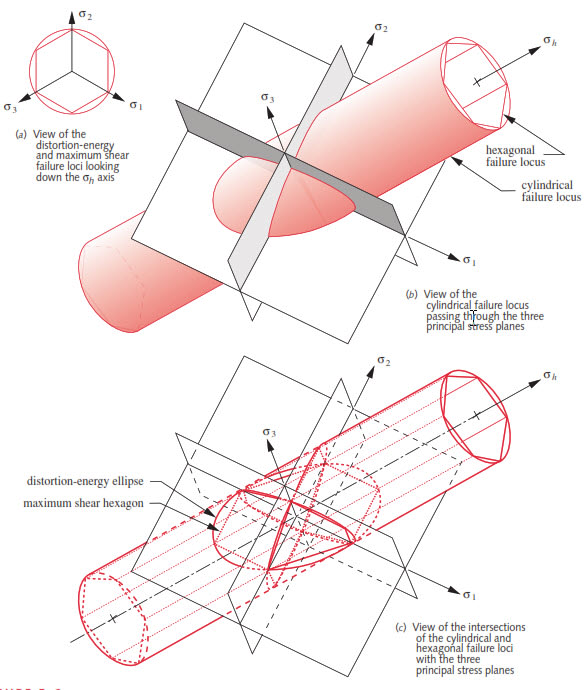
The question I have is, what’s the difference between von Mises, Tresca, and Principal Stress theory, and similar theories for that matter in the general sense (FEA or hand analysis)? Are there different names for these (ie max distortion energy = von Mises, max shear stress = Tresca)? Is there a way to down select which failure criteria one should use to calculate the margin of safety? Also, when can one use Ftu vs Fty for these failure modes in aerospace stress analysis? Aerospace typically calculates margins against Ftu (ultimate loads ~ 1.5 limit loads). Do you guys recommend any sources that go over this which would clarify why I was applying max principal stresses for a ductile material?
Even though I’m not an expert in composites, failure modes make more sense when dealing with composites in aerospace. For example, maximum strain criteria use over max stress criteria because the homogenized ply strains have determinate correlation to fiber/matrix phase in the fiber direction. The use of Tsai-Wu criteria over Tsai-Hill also makes sense as Tsai-Wu considers both tension and compression stresses. However, the use of linear criteria (max strain criteria specifically), is simpler to use, more closely related to the physics, and accurate enough.
I'd really appreciate any information. Thanks in advance!
The reason why I ask is because I've mostly worked in aerospace and I first started my career out of college as a stress engineer at a small company. I used extensive FEA to perform static and dynamic analysis and applied von Mises stress criterion to calculate MS against Ftu material allowables (metallic structures steel, aluminum and Inconel). Then I worked performing stress analysis of airframe fuselage metallic (aluminum) repairs for an OEM and used max principal stresses to compare against Ftu in MMPDS to calculate margins of safety. General guidelines suggest using von Mises for ductile materials, not max principal stresses, but I used principal stresses in this case.
After some time, I spent the majority of my career as a designer and leading up to this I didn't question the senior stress engineers in my short stint as a stress engineer. When I returned to stress analysis, I worked on nacelle repairs and, in one occasion, I had to calculate the margin of a blended metallic fitting, which was certified using FEM in the SCN using von Mises stress criterion. Due to limited data, my approver said it would be sufficient to compare the change in area and apply a knockdown factor to the FEM data and refer to Fty (yield), not Ftu (ultimate), due to the use von Mises stress during certification. That made me become curious about different failure modes but whenever I questioned things with other engineers I only seem to get in trouble as I discredited them from doing things the right way.
The question I have is, what’s the difference between von Mises, Tresca, and Principal Stress theory, and similar theories for that matter in the general sense (FEA or hand analysis)? Are there different names for these (ie max distortion energy = von Mises, max shear stress = Tresca)? Is there a way to down select which failure criteria one should use to calculate the margin of safety? Also, when can one use Ftu vs Fty for these failure modes in aerospace stress analysis? Aerospace typically calculates margins against Ftu (ultimate loads ~ 1.5 limit loads). Do you guys recommend any sources that go over this which would clarify why I was applying max principal stresses for a ductile material?
Even though I’m not an expert in composites, failure modes make more sense when dealing with composites in aerospace. For example, maximum strain criteria use over max stress criteria because the homogenized ply strains have determinate correlation to fiber/matrix phase in the fiber direction. The use of Tsai-Wu criteria over Tsai-Hill also makes sense as Tsai-Wu considers both tension and compression stresses. However, the use of linear criteria (max strain criteria specifically), is simpler to use, more closely related to the physics, and accurate enough.
I'd really appreciate any information. Thanks in advance!

![[lol] [lol] [lol]](/data/assets/smilies/lol.gif)
![[wink] [wink] [wink]](/data/assets/smilies/wink.gif) You are right though, without internal loads data a comparative analysis is the way to go, once the load paths are understood. Maybe I was taking things a bit too literally with the area comparison comment, but my point is... that is far too simplistic, even for a repair analysis without OEM data. A relative analysis can be pretty detailed. The problem is if you simply compare area, you are inherently assuming that the stresses are proportional to area. But (and I know you guys already know this, but to illustrate my point):
You are right though, without internal loads data a comparative analysis is the way to go, once the load paths are understood. Maybe I was taking things a bit too literally with the area comparison comment, but my point is... that is far too simplistic, even for a repair analysis without OEM data. A relative analysis can be pretty detailed. The problem is if you simply compare area, you are inherently assuming that the stresses are proportional to area. But (and I know you guys already know this, but to illustrate my point):