Calculate Section Modulus for an Irregular Section - (using X, Y Cordinates) 7

[DimzK]

Hi all,

I have a simple mechanics of solids type question which I have been googling, but I have not been able to find an answer.
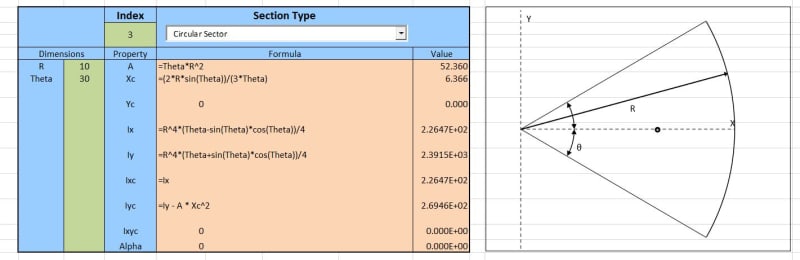
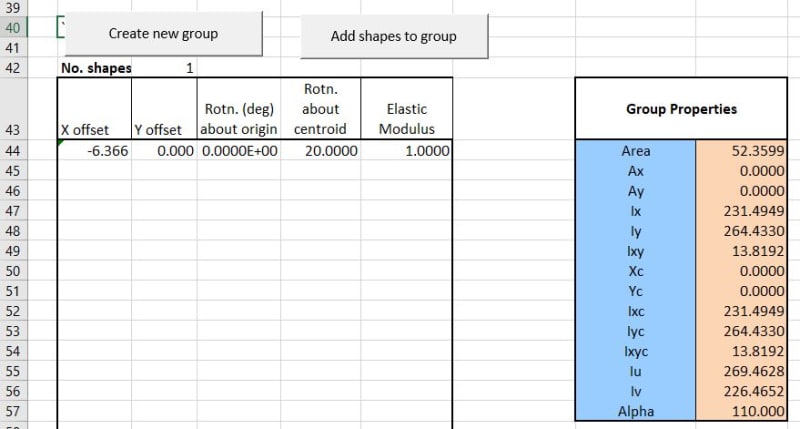
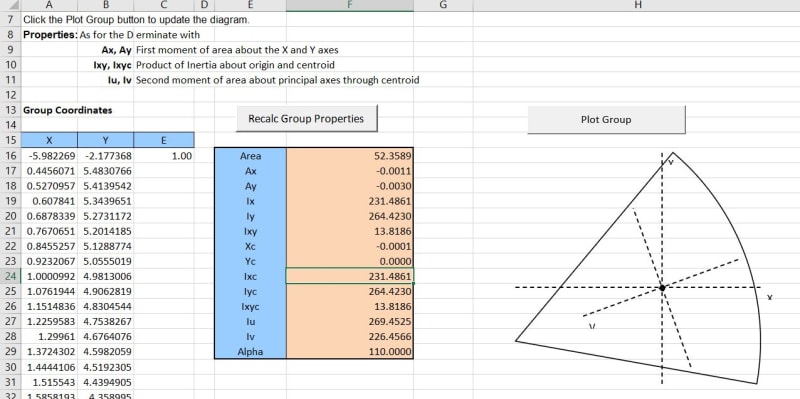
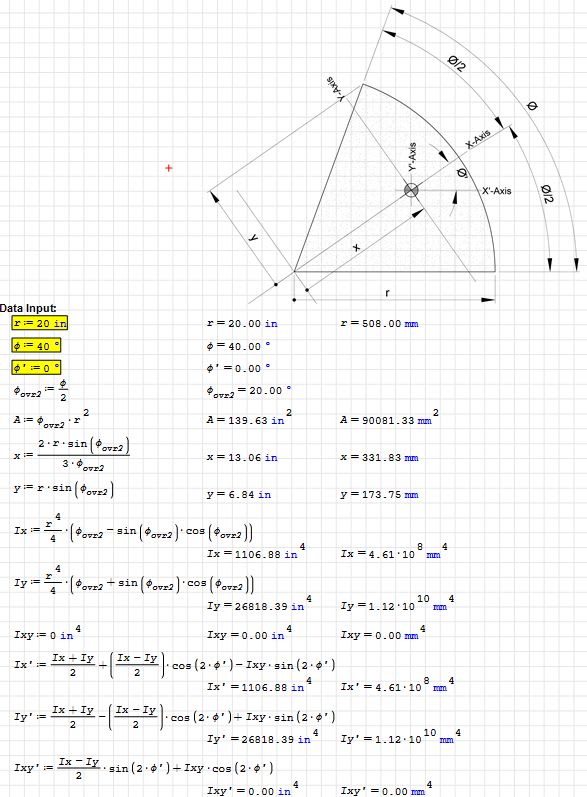
I have an irregular shaped section with bending moments imposed about its X axis as shown below.

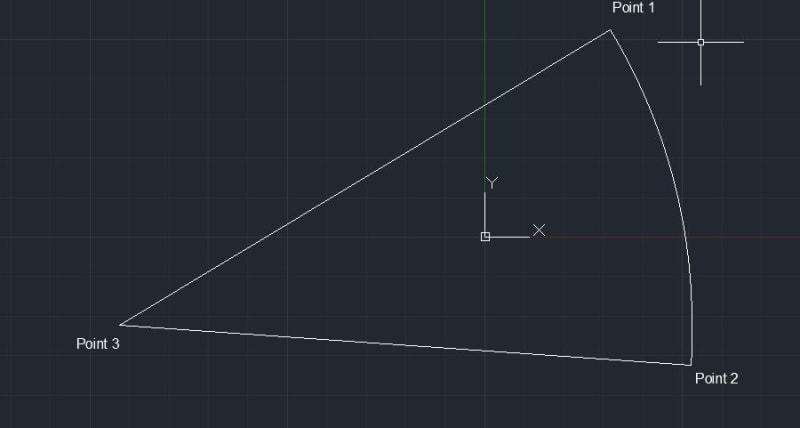
The centroid of the shape coincide with the x=0 aand y = 0 of the co-ordinate system.

Now for me to check my bending stresses at point 1 , 2 and 3, I need calculate my section modulus about Section X for each of these points.
If this was a simple rectangle, we could simply use B * H^2/6.

Is there any formular which would let me calculate the corresponding section modulus for these three points if I provide co-ordinates and Ix, Iy and Ixy values which I cam get of Autocad.

For context, I am trying model a deteriorated timber pile to check load capacity.

Any help would be appreciated.

Cheers,
Dimuth

 

[dik]

Can you find the results for a segment, with the centroid located parallel to the outer part of the circle, and rotate the values? I seem to recall there was a transformation to the I value based on rotation...

Rather than think climate change and the corona virus as science, think of it as the wrath of God. Feel any better?

-Dik

 

[dik]

Here's the first part...

and maybe the second...

I think that will do it... just a matter of putting the stuff together, I think. That goes back so far, it makes my head hurt... over 50 years. Having the new moments of inertia, it's a matter of determining the Section Modulii. Let me know if it works.

Rather than think climate change and the corona virus as science, think of it as the wrath of God. Feel any better?

-Dik

 

[IDS]

My spreadsheet at:

https://newtonexcelbach.com/2018/06/24/using-section-properties-group/

will find section properties for a variety of defined shapes.

The standard shape for a circular segment is symmetrical about the x-axis, but the group feature allows you to rotate any shape about the origin, which should give the results you need. The link above gives an example of rotating a circular segment through 90 degrees, but you can use any angle.

Alternatively (and the way I'd probably go) you can define the shape with coordinates and get the section properties for any shape.

Doug Jenkins
Interactive Design Services

http://newtonexcelbach.wordpress.com/

 

[DimzK]

Thanks Dik, for your input, I am still missing the vital bit I need to get the section modulus.
I may have to go for the old method of picking Ixx / distance from centroid to extreme comrpession/tension fiber, <- and guess the extreme points

 

[IDS]

Just tried the spreadsheet, and it seems to work OK:

On the DefShapes sheet, select "Circular Sector" and enter the radius and theta:

Scroll down to the Group properties input, enter -Xcentroid as "X offset" and the rotation angle, then click "Create New Group":

Go to the Coords_Group sheet and click Plot Group. You can then find the coordinates of the three corner points, with the origin at the centroid, which you can use to calculate the 3 section modulus values:

I have attached a copy of the spreadsheet. Please ask if any problems.

Doug Jenkins
Interactive Design Services

http://newtonexcelbach.wordpress.com/

 

[rb1957]

do you want a sector, which is easily done, or a generalised shape ?

you can always go back to the basic definition of moments of area ... intergral of xdA and integral of x^2dA.
or you can divide your shape into smaller standard pieces (squares and triangles) and sum.

another day in paradise, or is paradise one day closer ?

 

[dik]

If you have the point coords, you have the geometry. For that type of shape, I'd draw the object in autocad and measure the angles and dimensions. Draw it, find the centroid, draw the centroid, and take measurements, rotate it and take new measurements.

Rather than think climate change and the corona virus as science, think of it as the wrath of God. Feel any better?

-Dik

 

[rb1957]

CAD should do it all for you, no?

another day in paradise, or is paradise one day closer ?

 

[Denial]

You should probably also allow for the fact that, as per your diagram at least, your "pile" cross-section is not being bent about a principal axis.[&nbsp;] (Inverted commas because that's an impressive amount of deterioration you seem to be showing.)

 

[dik]

Thanks, Doug...

Rather than think climate change and the corona virus as science, think of it as the wrath of God. Feel any better?

-Dik

 

[JStephen]

In addition to the other solutions noted, numerical integration via spreadsheet is fairly easy to whip up. Presumably, you don't know the geometry to 4 decimal places anyway, so it wouldn't be hard to get close enough.

 

[jec67]

Am I missing something? You state you have Ix and Iy. The stress at a given point for a moment about the x-axis is then Fb=(M Y)/Ix. Fb is the stress, M is the moment, Y is the perpendicular distance from the X-axis to the point under consideration.

 

[Celt83]

Jec67:
That formula is only correct for symmetric/principle axis bending, when Ixy=0.

My Personal Open Source Structural Applications:

https://github.com/buddyd16/Structural-Engineering

Open Source Structural GitHub Group:

https://github.com/open-struct-engineer

 

[jec67]

So then it’s a matter of determining the moments of inertia about the principal axes per DIK’e post and resolving the moment to the components about the principal axes, no?

 

[dik]

I'm pretty sure of it... and then S can be determined by the x and y distance to the outside fibre. If I'm bored tomorrow, I'll do up an SMath program. Nearly finished my lag screw one, but want to check the output with an actual connection design program. I don't have one, but my colleague Vic has one... he's the wood expert I use.

Rather than think climate change and the corona virus as science, think of it as the wrath of God. Feel any better?

-Dik

 

[dik]

Something like (ERROR: Iy HAS TO BE TRANFORMED):

[URL unfurl="true"]https://res.cloudinary.com/engineering-com/raw/upload/v1637463102/tips/Moment_of_Inertia-Segment_prsdxc.sm[/url]

Rather than think climate change and the corona virus as science, think of it as the wrath of God. Feel any better?

-Dik

 

[BAretired]

I[sub]xy[/sub] = 0 is surprising!

Edit: See below!

BA

 

[dik]

I guess the formula's correct... I didn't check the derivation... just plugged numbers into a formula... and if you set the angle of rotation equal to 0, you get the same values for no rotation... something seems to be OK.

Rather than think climate change and the corona virus as science, think of it as the wrath of God. Feel any better?

-Dik

 

[BAretired]

I spoke too soon. It is not surprising, because the shape is symmetrical about the X axis. Sorry about that, dik.

BA