Consequences of increasing the length of the iron core in electric motor 7

[EngRepair]

Hypothetical, theoretical question about electric motors.
Let's say we have a fully functional three-phase squirrel-cage LV motor.
Let's imagine we made another one with exactly the same geometry of stator and rotor lamination, exactly the same winding (turns/coil, wire size, pitch, etc...).
The only difference should be the length of the stator and rotor cores.
Let's say the length is increased by 10%.
Also, the motor load will remain the same as before.
What changes will this cause in terms of hp, torque, rpm, FLA, NLA, efficiency, and power factor?
It would be greatly appreciated to hear some expert opinions.

 

[electricpete]

I have to apologize for putting words in Bill's mouth by interjecting the synchronous machine equation. (Sorry Bill!)

He was probably envisioning an equation similar to that found in Krause.

If that is the case, then hopefully my last post would serve to show that my equation is saying the same as Krause's equation that he is familiar with.

 

[electricpete]

I'll see if I can make a vector diagram corresponding to the conclusions of my posted date 21 Mar 23 20:46 comparing my equation to Krause's. The proof of the equivalent form looks correct to me (and using the vector cross product eliminates a heckuva lot of trigonometry) but to this point I'm having a hard time building the entire vector diagram.

 

[waross]

I am sorry, edison123

I fully stand by my assertion that torque is proportional to the product of flux density B and the motor current regardless of motor core length and dia.

I don't do equations anymore, if I can avoid it, but I always looked for the basics behind the equations.
I remember the basic relationships very well.
Your statement is wrong on two points.
A given flux density will exert a force PER UNIT LENGTH on a conductor. LENGH MATTERS.
Torque is dependent on the tangent force on a conductor and the radius from the center of rotation.
Thus, DIAMETER MATTERS.

--------------------
Ohm's law
Not just a good idea;
It's the LAW!

 

[electricpete]

I 100% agree with Bill on that. As I mentioned, if D and L played no role in torque production (for a given current turns and flux density), then everyone would make really tiny motors. If you don't want to believe the theoretical arguments presented then at least that should make some intuitive sense.

For op that is an important takehome (along with other items the torque speed curve may be lowered which can affect start performance and overload performance but not hp rating).

Bill and I are working through a minor disagreement and I know that contributes to the messiness of this thread but it shouldn't take away from the above important points for op.

I will continue my discussion with Bill in my next post...

 

[electricpete]

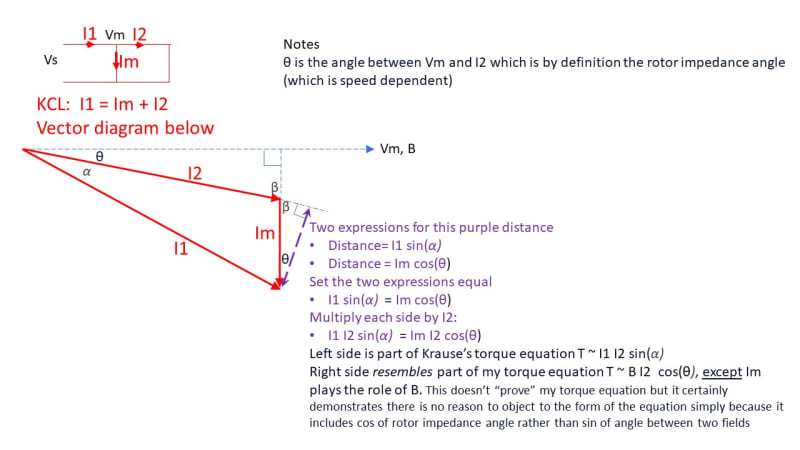
Attached is a vector diagram which proves that I1 I2 sin (alpha) = IM I2 cos (theta)
WHERE
[ul]
[li]alpha is angle between I1 and I2[/li]
[li]theta is angle of the rotor impedance (which is the angle between Vm voltage and I2 current).[/li]
[/ul]

Left side is part of Krause’s torque equation T ~ I1 I2 sin(α)
Right side resembles part of my torque equation T ~ B I2 cos(θ), except Im plays the role of B.

This doesn’t fully “prove” my torque equation is the same as Krause's, but it certainly demonstrates there is no reason to object to the form of my equation simply because it includes cos of rotor impedance angle theta rather than sin of angle between two fields alpha. The fact that B is not present in the result is expected given the circuit used (B is not a vector within this circuit although we know its angle matches Vm). To use the results of the vector diagram to generate full proof that my torque equation represents the same thing as Krause's torque equation would require careful examination of the other constants used in the full equations... I suspect that can be done but I don't foresee myself spending the time to attempt that. So I will leave it there (*)

I would also note the left side does have an intuitive appeal as alignment of magnets, while the right side would have intuitive appeal as Lorentz force which acts at radius R to produce torque if we substitute B for Im.

Going back to my earlier post 21 Mar 23 20:46 using vector cross product I now think it was correct in certain respects and incorrect in certain respects:
[ul]
[li]It was correct to easily demonstrate I1 I2 sin (α) = Im I2 sin(β) where α is I1 I2 angle and β is Im I2 angle (this is exactly the same as the conclusion of the vector diagram noting that β and θ are complimentary and therefore sinβ =cosθ... but we had reached that conclusion with a few simple cross products rather than all that complicated vector diagram and trig!). That in itself is an interesting transformation and explains some of the variability in which variables appear in textbook torque equations (some have I1 and I2 and some have Im and I2). [/li]
[li]It was not correct to conclude that alpha was related to theta by a pi/2 factor. Theta does carry similar information as alpha in terms of the angle whose sin is needed to compute torque from two current (or field) quantities, but the selection of currents is different and the angles don't have the simple pi/2 relationship that I suggested.[/li]
[/ul]

(*) Why am I leaving it there? I don't believe my torque equation requires proof from the vector equation since my torque equation was already proven by direct comparison to Liwschitz Garik textbook with copy of relevant page attached to my post 21 Mar 23 02:34 (if anyone wants me to walk through an algebraic proof that they're the same, let me know). Also the logic for the equation was clearly shown in an earlier linked paper posted 7 Mar 23 14:21. Being intimately familiar with that paper I firmly believe it's a proof, and it's really simple to understand if you accept the use of Lorentz for this purpose, but you might have to invest some more time to understand why that is an appropriate approach given that the conductors are shielded from airgap flux by the slots, and the force acts primarily on the core.... there are many different proofs of this given in the paper and also excerpts from many texts in the back of the paper which attest that using the force on conductor equation along with Bgap gives the correct result. The paper also includes calculations on an example motor which matched the hp rating pretty closely (based on FLA adjusted for number of parallel circuits, nameplate speed, and the other equation variables B, N, L, R). If anyone has data for a motor they want to try it out on, feel free to post motor details and we can try it out on your motor.

 

[waross]

Sorry, Pete.
I did not realize that your angle was related to the impedance of the rotor field.
I hope that resolves our disagreement.

On a completely different topic.
The diagram that you posted in your last post triggered memories of my first power factor survey.
Working for a contractor, a power factor issue came through the door and was dropped on my desk.
We had ammeters, voltmeters and meggers, but nothing to measure power factor.
We also had a couple of PF correction capacitors in the shop.
Now, I did this once and it was a valuable learning experience.
The method remained in my mental tool box as a quick way to determine the PF of a motor, but I found a much better way to correct PF for a large plant.
I connected about 8 feet of flexible cord to the PF cap, with alligator clips on the end of the cable.
Then I would start the motor and record:
The capacitor currnt,
The uncorrected motor current,
and
The corrected line current.
Back in the office, I laid out a vertical line on the drafting table to represent the capacitor current.
Then with a compass I laid out the line current and the motor current to form a triangle similar to the triangle in your last post.
Then, using the drafting machine, a horizontal line from the point of the triangle and intersecting an extension of the vertical line gave me a scale of the real current.
Real current divided by the motor current (both scaled from the drawing board) gave me the PF.
Thanks for the reminiscence, Pete.
ps; Then I had an epiphany.
For plant PF correction, the information was already available, at revenue accuracy.
I would find the accounts payable clerk and request copies of the last two years power bills.
If a PF penalty is charged, the monthly KVARHrs are shown on the power bill.
Divide each months KVARHrs by the hours in the billing period.
Throw out two or three high outliers.
Connect enough KVARHrs of correction to correct to 100%.
Penalties started at 90% and that 10% grace will take care of the outliers.
Then came the art of installing the caps.
A favoured connection was 200% correction on large motors.
That left the overload settings the same and corrected for a host of smaller motors.
There were a few other tricks.

--------------------
Ohm's law
Not just a good idea;
It's the LAW!

 

[electricpete]

No worries.

Before we leave the subject of the torque equations, I’d just like to revisit the two different forms of torque equations that involve sin of an angle. I’d call one the Krause torque equation and one the synch-only torque equation:
[ul]
[li]The one I call the synchronous-only torque equation is T = |V1||V2|sin(delta)/(w*X). It involves the angle delta across a reactance (usually synchronous reactance). It’s not often applied to other machine types and never to induction machines to my knowledge. This sync-only equation does impose a maximum torque (when delta hits 90).[/li]
[li]The one I call the Krause equation is T ~ I1 I2 sin(alpha). It roughly corresponds to the interpretation as 2 electromagnets with an angle alpha between them. It can apply to many types of machines (including induction motors) with suitable choice of fields or currents. It does not impose any maximum torque on the induction motor… as you increase the load the endpoints of the vectors I1 and I2 on my diagram will move mostly to the right and slightly down but the vertical distance between the endpoints would decrease slightly... so if anything the angle alpha might get a bit smaller as the load increases. Of course we do have a maximum torque on the torque speed curve attributable to impedance magnitudes and angles that both vary with speed, but it’s not tied to the angle alpha reaching 90.[/li]
[/ul]I’m pretty sure you understand all that but I felt it was worth summarizing the distinction before moving on. If nothing else it'll help me remember.

 

[electricpete]

The one I call the Krause equation is T ~ I1 I2 sin(alpha). It roughly corresponds to the interpretation as 2 electromagnets with an angle alpha between them. It can apply to many types of machines (including induction motors) with suitable choice of fields or currents.

One thing to add, per the vector diagram above we have 3 different expressions that are equivalent:
[ul]
[li]T ~ I1 I2 sin(alpha) = Im I2 sin(beta) = Im I2 cos(theta)[/li]
[li]...where alpha and beta are angle between the two currents in their respective expressions... and theta is the speed-dependent rotor impedance angle[/li]
[/ul]

… as you increase the load the endpoints of the vectors I1 and I2 on my diagram will move mostly to the right and slightly down but the vertical distance between the endpoints would decrease slightly... so if anything the angle alpha might get a bit smaller as the load increases. Of course we do have a maximum torque on the torque speed curve attributable to impedance magnitudes and angles that both vary with speed, but it’s not tied to the angle alpha reaching 90.

It's interesting that the angle alpha decreases with increasing load. At first glance that seems counterintuitive if you're visualizing something like two permanent magnets. But of course we have something more like two electromagnets whose strength can increase, so the angle is not the whole picture.

So I wanted to do a sanity check to see if this decreasing angle plausibly explains a test case. The test case I looked at is neglibly small leakage reactances. It's not necessarily realistic but it's easier to analyse to see if the results make sense. In particular I wanted to see if the model predicts that at high load the current will approach a linear relationship with torque. With the negligble leakage reactances then the endpoints of I1 and I2 are moving purely horizontal to the right and the angle alpha clearly gets smaller. So does T~I1 I2 sin(alpha) become linear with current? We know alpha is decreasing but I1 and I2 are BOTH increasing, so at high load torque approaches something like alpha * I1 * I2, so the coefficient of I1 is alpha times I2 where alpha is decreasing and I2 is increasing. Is that approaching linear (constant coefficient of I1)? I have no idea....

...BUT if I simply look at the equivalent form T ~ Im I2 sin(beta) in the same scenario (low leakage reactance, increasing load, the endpoints of I1 and I2 vectors move straight to the right), we see beta approaches 90, sin(beta) approaches 1, we know Im is relatively constant (low leakage reactance) so it's easy to see with this version of the formula that this model predicts torque will approach a linear relationship with I2 at high loads. And since I2 approaches I1 at high loads, we also conclude linear relationship of torque vs I1 at high loads (as expected).

So in my mind, the equations do what I expect them to do in this test case (which is not necessarily representative of a real motor). And it's handy to have multiple forms of the equation.

(I probably could have concluded that the angle alpha between rotor and stator current goes down with increasing load by noting that any difference in angle is attributable to magnetizing current in the stator branch, whose contribution diminishes relative to load current as we increase the load current, but I wanted to explore the use of these torque expressions)

 

[waross]

10% increase in core length = 10% increase in output, torque, NLA, FLA and iron loss.

No. of turns must change proportionately for the above parameters.

May I restate that;
10% increase in core length plus "No. of turns must change proportionately" = 10% increase in output, torque, NLA, FLA and iron loss for the above parameters.

I see the corollary as;
10% increase in core length and no change in turns does not equal a 10% increase in output, torque, NLA, FLA and iron loss for the above parameters.

Why do the formulae not agree?
A formula may simply show a relationship between parameters, with the understood caveat of "All else being equal".
You may show a formula that correctly describes the relationship between flux density and torque, with the understanding that any parameters not included must remain equal.
Once you consider the full formula, with no unspoken caveats, you will see that conductor length does enter into the equation and there is no loss of torque.
I will admit to 10% greater I² losses.
I suggest that the modified motor will be better able to withstand a higher supply voltage before saturation, and thus may be better suited to rural pump use.


--------------------
Ohm's law
Not just a good idea;
It's the LAW!

 

[electricpete]

For anyone paying attention to my vector diagram, I think I need to revisit the polarities of my variables. I made a choice to label I2 polarity such that I1=I2+Im. But there is another choice to label the polarity of I2 going left towards the Vm node, such that I1 + I2 = Im. It would change the diagram quite a bit.

At any rate for this thread I want to caution anyone to be careful relying on my diagram. I'm reasonably sure that a revised vector diagram would still support the same conclusion that T ~ I1 I2 sin(alpha) = Im I2 cos theta (it can't be a coincidence that my diagram supported that). That was the main thing I was trying to do with the vector diagram.

 

[waross]

I can't count the number of times that my "yondering" has found me in a remote location, with no textbooks and little test equipment available to solve a problem.
I have had, many times to depend on my memories of basics, logic and ingenuity to solve a problem.
I have wandered from a village in the Yukon Territory in the north to Honduras in the south.
From Vancouver Island in the west to Darlington Nuclear plant in Ontario in the east.
I have solved problems in the jungle of the Moskito Coast One fellow from down the coast sent a couple of men to collect me to look at his generator.
They took me across a lagoon in a dugout canoe. Then we transitioned to horse back and travelled down the beach to his village.
I solved a generator issue near the shore of Lac Labarge in the Yukon.
(It was on the marge of lac LaBarge that I cremated Dan MacGee. Robert Service.)
Life has been a lot of fun.
My latest adventure has been driving a diesel, dually, one ton from Alberta Canada to Indiana and pulling large, new travel trailers back to dealers in Western Canada.
I finally had to admit that I was getting too old and quit that job in January.
I did about 30 trips.

--------------------
Ohm's law
Not just a good idea;
It's the LAW!