Flexural Strength of a Steel Plate 2

[gmoney731]

I have a 1/2" cantilevered steel plate welded to a steel beam. The plate is experiencing a 13 kip load as shown in the image below:

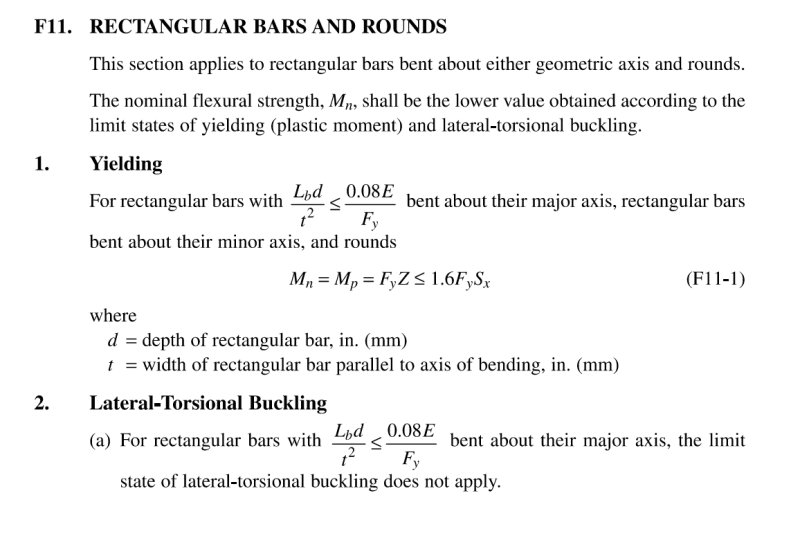
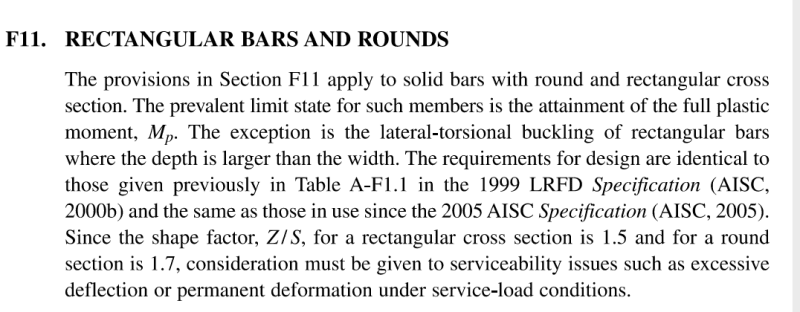
My question is, can I use AISC 14th F11. RECTANGULAR BARS AND ROUNDS and apply those equations to calculate flexural strength? My understanding was that bars are different than plates, even though they both have rectangular sections.

Can anyone provide structural theory that can justify it? I have seen some explanations on here talking about the Z/S ratio.

At work, I am being told to just check (phi)Mn = Fy*S, and I also want to know whether it is reasonable to assume that the plate can get to the plastic moment without buckling or other modes of failure?

 

[KootK]

To dial it back to steel for a moment, this is from Akbar Tamboli's Handbook of Steel Connections. This section was authored by Larry Muir and Bill Thornton who, as we know, are really just fringe element nobodys when it comes to steel connection design. These three paragraphs are basically the framework upon which steel connection design is built.

 

[centondollar]

"In all of the STM design examples that I've reviewed from ACI, FIB, Schlaich, numerous US DOT's, and others I have not once seen anyone attempt to "converge" an STM model. It seems to be you and you alone who feel that is necessary. I believe that an STM model requires no "converging" because:"

The accuracy of STM depends on how well you estimate equilibrium with the truss (bar) model, and thus, any configuration will not suffice.

"1) STM models are in equilibrium with their external loads by definition."
This is not strictly speaking true. Imagining three bars in a deep beam loaded by mid-span point load does not mean that those bars exist and that equilibrium is achieved. The same goes for more complicated structures.

"2) Any approximations made in the development of STM models are well within the range of normal engineering approximation."
That depends entirely on how well the engineer mimics an elastic (or otherwise uniquely determined) stress field.


"3) STM is a lower bound method."
You write this, but it does not make it so.

"Can you point to any example that we might review in which someone has attempted to "converge" and STM model? We pretty much always attempt to have our STM models mirror elastic stress distributions but that's done at the beginning of the design process and is not at all iterative."
The strain energy of the STM should equal the strain energy of the unique (elastic or by other means derived) equilibrium solution.

"I submit that no one is "converging" STM models because they don't require converging."
The correct statement is that the STM, like many other things in engineering, is surrounded by misunderstanding.

 

[centondollar]

"Ductility is, in fact, very easy to measure. The trick is that you measure it in a laboratory setting. Then you use the results of that laboratory testing to inform the simplified design procedures used by engineers in practice so that they are not stuck with the onerous task of somehow trying to calculate how ductile a thing is on the fly. This process is basically what allows us to have "connections" in all of our favorite materials."
The words "easy" and "experiment" do not go together, and experiments do not always provide results that can be extrapolated to more complicated situations.

"I presume that you are aware of the significant amount of testing that has be done to validate the strut and tie method of concrete design over the years? Many of the code provisions that govern strut and tie design exist, in part, to ensure that the members possess enough ductility for the STM method to be valid. This includes things like:"
I know that the STM (which, by the way, is at the core of the shear design model you posted earlier) cannot properly predict slender RC beam or plate behavior.

 

[centondollar]

"To dial it back to steel for a moment, this is from Akbar Tamboli's Handbook of Steel Connections. This section was authored by Larry Muir and Bill Thornton who, as we know, are really just fringe element nobodys when it comes to steel connection design. These three paragraphs are basically the framework upon which steel connection design is built."
No gaps and tears, he writes. Satisfying equilibrium, he writes. Those are exactly requirements that many STM models (not all, mind you) do not fulfil.

I do not object to the statements you keep quoting (I too have learned this in university), but to the way you interpret them. Equilibrium (from e.g., elastic solutions) cannot be satisfied for a complicated geometry (the stress field for a simple 2D membrane in-plane stress problem can be quite complicated) and loading if one uses a strut-and-tie model with bars so few and regularly spaced that it looks like an ordinary steel truss. Capturing the equilibrium for any non-trivial problem is hard, and - if done in the scientific way - requires comparison of strain energy of the known unique (e.g., elastic) solution and the strain energy of the proposed STM.

 

[Tomfh]

I imagine this approach would be lower-bound (same as for concrete), but maybe I'm wrong.

Is it really lower-bound to assume a pin restraint along the edge of your compression strut? That hypothetical strut is restrained by a spring along its edge, not a hard pin. If you deleted the pin and considered the strut a free cantilever (i.e. effective length k=2), then yeah, lower bound.

 

[bugbus]

Tomfh, I agree with that completely. The pin was a pretty crude assumption. Ignoring that, it should be lower bound.

 

[KootK]

The pin was a pretty crude assumption.

I didn't even notice the pin. That's what I get for Eng-Tipping on my phone. This is the STM model that I favor and mistakenly thought that you had originally proposed. The vertical is fixed for moment out of page (k=2) and pinned for moment in the plane of the page. I was too lazy to attempt an isometric.

 

[KootK]

"1) STM models are in equilibrium with their external loads by definition."
This is not strictly speaking true. Imagining three bars in a deep beam loaded by mid-span point load does not mean that those bars exist and that equilibrium is achieved.

It doesn't mean that those bars do exist and are in equilibrium but, rather, that they could exist if they need to and would be in equilibrium in that that. And that is what matters.

"2) Any approximations made in the development of STM models are well within the range of normal engineering approximation."
That depends entirely on how well the engineer mimics an elastic (or otherwise uniquely determined) stress field.

So do it well then. That's the engineering part of the exercise. As many test validated STM models have proven over the years, skilled engineers have little difficulty in developing STM models that perform well in this regard.

"3) STM is a lower bound method."
You write this, but it does not make it so.

My saying it doesn't make it so.
Everyone on planet earth who has ever written about STM (other than you) saying it... makes it highly, highly likely.

"Can you point to any example that we might review in which someone has attempted to "converge" and STM model? We pretty much always attempt to have our STM models mirror elastic stress distributions but that's done at the beginning of the design process and is not at all iterative."
The strain energy of the STM should equal the strain energy of the unique (elastic or by other means derived) equilibrium solution.

That is false as a lower bound method will never, by definition, have it's strain energy perfectly match that of the true, elastic solution.

I note that you have conveniently disregarded my request for any printed example of anyone ever having attempted convergence in STM modeling.

"I submit that no one is "converging" STM models because they don't require converging."
The correct statement is that the STM, like many other things in engineering, is surrounded by misunderstanding.

It seems to me that, when it comes to STM, the misunderstanding is predominantly yours. There exists a veritable army of researchers, authors, and practitioners who clearly feel that their understanding of the strut and tie method justifies their skillful use of it.

 

[KootK]

"Ductility is, in fact, very easy to measure. The trick is that you measure it in a laboratory setting. Then you use the results of that laboratory testing to inform the simplified design procedures used by engineers in practice so that they are not stuck with the onerous task of somehow trying to calculate how ductile a thing is on the fly. This process is basically what allows us to have "connections" in all of our favorite materials."
The words "easy" and "experiment" do not go together, and experiments do not always provide results that can be extrapolated to more complicated situations.

So what, then? Are we just going to stop trusting industry research to inform design practice altogether? How are we to design anything at all if that is to be the case? Rationally, industry testing should be the gold standard when it comes to informing design practice.

"I presume that you are aware of the significant amount of testing that has be done to validate the strut and tie method of concrete design over the years? Many of the code provisions that govern strut and tie design exist, in part, to ensure that the members possess enough ductility for the STM method to be valid. This includes things like:"
I know that the STM (which, by the way, is at the core of the shear design model you posted earlier) cannot properly predict slender RC beam or plate behavior.

STM informs the shear truss model. However, the shear truss model is far cry from being as sophisticated and complete a design method as is STM. As such, it is invalid for you to claim that, because we're moving away from the shear truss model, STM is somehow flawed. In that, it is your logic that is flawed.

 

[Enable]

Thanks for the recommendations KootK much appreciated.

 

[KootK]

No gaps and tears, he writes. Satisfying equilibrium, he writes. Those are exactly requirements that many STM models (not all, mind you) do not fulfil.

I submit that those requirement would indeed be met by virtually all of the rather pedestrian STM's that I've seen you object to over the last couple of months. In my opinion, you are spuriously deterring people here from attempting to make use of strut and tie design procedures in situations where they are widely deemed to be appropriate.

I do not object to the statements you keep quoting (I too have learned this in university), but to the way you interpret them. Equilibrium (from e.g., elastic solutions) cannot be satisfied for a complicated geometry (the stress field for a simple 2D membrane in-plane stress problem can be quite complicated) and loading if one uses a strut-and-tie model with bars so few and regularly spaced that it looks like an ordinary steel truss.

In this thread, you have objected to the use of a two member truss inside a square plate made of one of the most ductile materials used in construction. A two member truss! You consider that to be too "complicated" of a geometry for a skilled engineer to prosecute successfully? What could possibly be simpler than this situation?

 

[TLHS]

I have definitely used the 'ignore parts of the plate to treat it as a truss with simple buckling assumptions' method for things like this before.

There could be arguments about ways in which similar methods could be non-conservative because there are considerations like internal force transfer that they may not account for, or that there are inherent stress/strain compatibility assumptions that may not be valid. It doesn't seem like a thing here. However, this isn't the same as being an upper bound solution.

Strut and tie, or this type of ad-hoc lite version of strut and tie seems to be pretty definitively not upper bound. An upper bound method will have potential solutions for capacity that are at the critical load or above it. It shows an upper bound for the potential capacity solution. The critical load is the minimum possible solution. No valid solution or model would have a lower capacity than the collapse load. It seems pretty trivial to prove that strut and tie is not an upper bound theorem and that you can make valid models that are below the failure load. This is also definitely the intent of the system.

Not being an upper bound methodology doesn't mean that there aren't ways to get non-conservative results from a lower bound methodology. There could be limits or assumptions that are invalid in certain situations, it could just be non-conservative for various reasons, and all sorts of other fun stuff. It's just not an upper bound theory.

 

[Tomfh]

Can someone please attach or summarise the F11 buckling method? Does it explicitly cover a situation like this, with a slender cantilever plate?

 

[KootK]

Here you go Tomfh. The section is meant to apply, generically, to beam-like members made from solid, rectangular sections.

 

[KootK]

 

[KootK]

You can actually have the entire standard for free: Link

 

[Tomfh]

Thanks!

 

[ALK2415]

you could simply trying a second solution
Box shape will always be stronger than flat plate
or latterly stiffened plates

 

[JLNJ]

I was looking at a plate last week by hand and instinctively used 1.5S for Z. I then looked it up and saw that it is 1.6S in the Spec. What not just say 1.5 for rectangular and 1.7 for rounds? This seems like an odd place to be stingy with paper and spec provisions. It also makes explaining things to a freshly-minted engineer more difficult. First I try to show them the logic for the derivation of a Spec provision, and then I can't explain what they put in the Spec, even for something as simple as S and Z of a rectangular bar.