Everydaylearning
Structural
Should there by sigma y and sigma z stresses in this plate due to poisons ratio effects? How would I go about finding exactly what they are?

Follow along with the video below to see how to install our site as a web app on your home screen.
Note: This feature may not be available in some browsers.

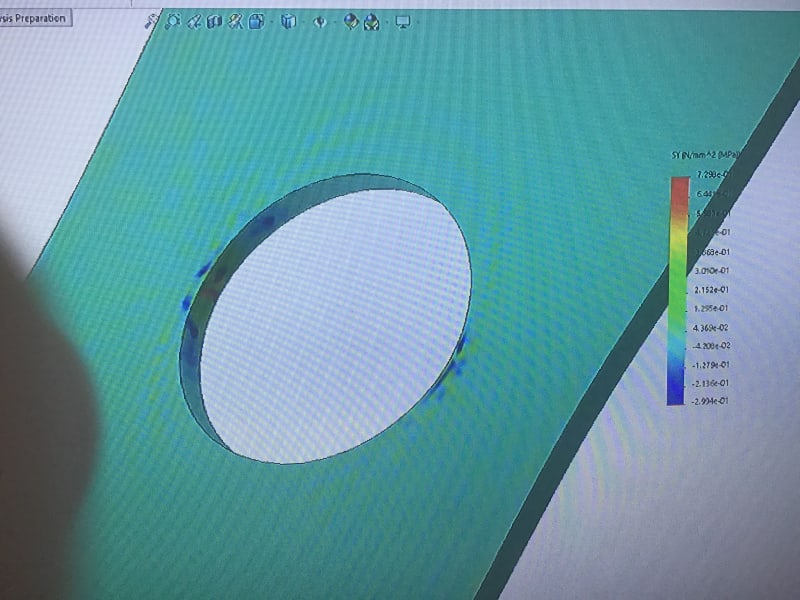

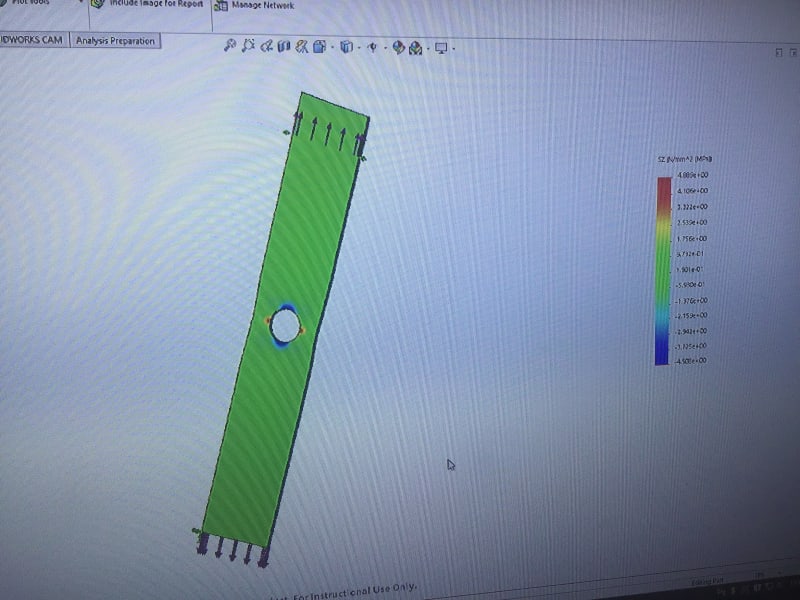
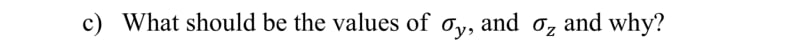
![[smile] [smile] [smile]](/data/assets/smilies/smile.gif) .
.
