Deension
Structural
- Oct 30, 2012
- 8
Hello Guys,
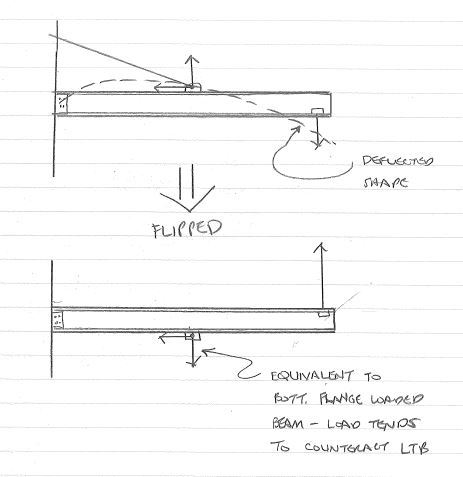
I am design a wall mounted jib crane (attached) that is in a triangular configuration. The Jib Arm is cantilevering about 17ft give or take; it is tied at about 13' with a tie 1" tie rod that is pin connected to the wall. The Jib is also pin connected to the wall. The angle between the jib and tie rod is about 10 degrees. My question is what k value (effective length factor) to us for design of the jib which is in signaficant compression and some moment.
I am teared between a k=1 for pin - pin condition and k = 2 for pin - free condition. I did come across a paper that uses k = 1 but I am not sure if I should use k = 1 or k = 2 for the designing the jib that is in compression. Any help from you guys would be greatly appreciated.
Best Regards
I am design a wall mounted jib crane (attached) that is in a triangular configuration. The Jib Arm is cantilevering about 17ft give or take; it is tied at about 13' with a tie 1" tie rod that is pin connected to the wall. The Jib is also pin connected to the wall. The angle between the jib and tie rod is about 10 degrees. My question is what k value (effective length factor) to us for design of the jib which is in signaficant compression and some moment.
I am teared between a k=1 for pin - pin condition and k = 2 for pin - free condition. I did come across a paper that uses k = 1 but I am not sure if I should use k = 1 or k = 2 for the designing the jib that is in compression. Any help from you guys would be greatly appreciated.
Best Regards