Alexey881
Mechanical
- Mar 24, 2013
- 18
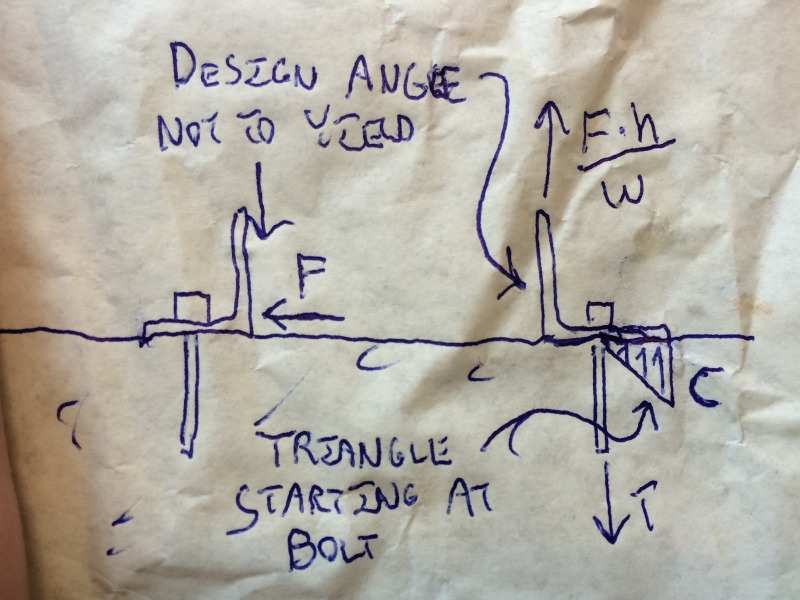
Hey everyone. How structural engineers would treat this problem if they had to determine all forces on all fasteners of a column loaded in the following way on top? (ignore weight)
h=w=10 in. a=b=1 in.
I've consulted several engineers, and each had a different way of resolving a force and of selecting a point of rotation, and in the end the answers of loads on the fasteners were very different. (some ways include prying, others don't) So what would you do?
h=w=10 in. a=b=1 in.
I've consulted several engineers, and each had a different way of resolving a force and of selecting a point of rotation, and in the end the answers of loads on the fasteners were very different. (some ways include prying, others don't) So what would you do?