FREE BODY DIAGRAM 1

[elinah34]

Hey,
I have a conceptual design, and I need to first check its potential strength.
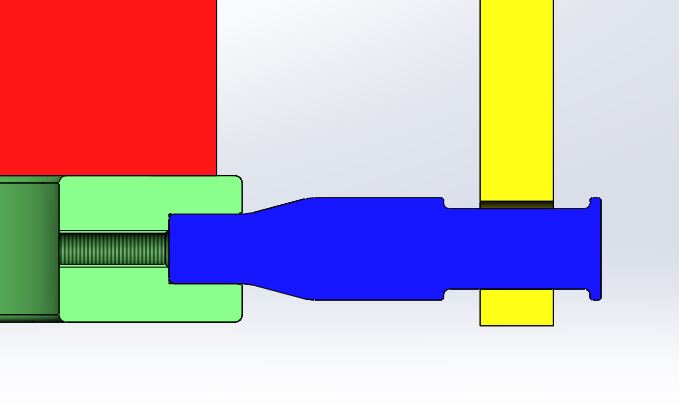
Here is a schematic picture of the conceptual design:

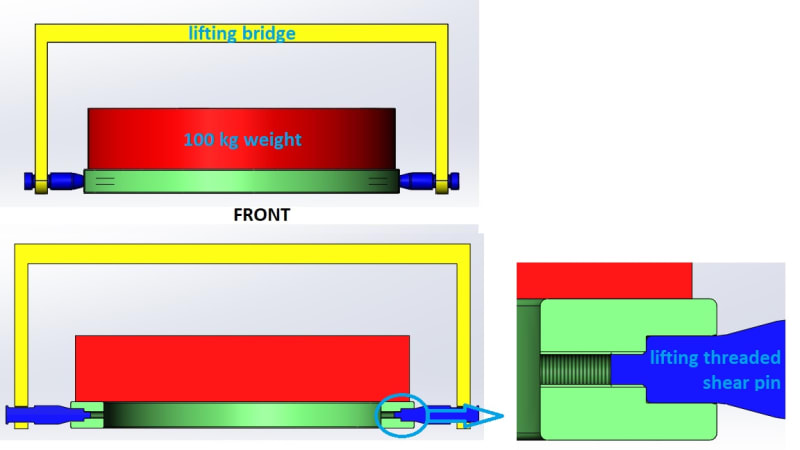
I have a lifting bridge for lifting the weight through its interface which has 2 Shear pins for taking the shear.
The Shaft/Hole fit (marked in purple in the picture below) is H7/g6, so there is a transition fit.

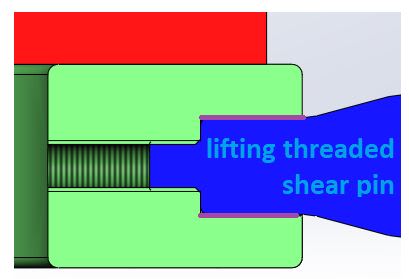
By the way, the thread is loose while the Shaft/Hole fit is tight, so theoretically the Shaft/Hole interface is the one to take the loads due to bending and not the thread, which is there only to axially secure the threaded pin in its place.
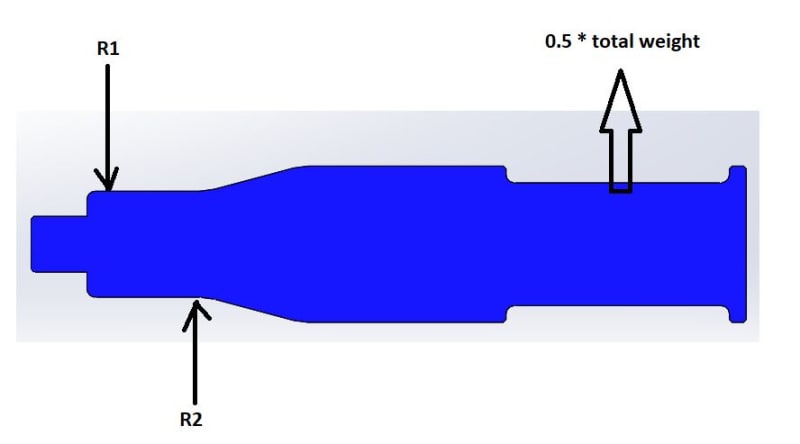
I tried to have a FBD (Free Body Diagram) of the threaded pin part, which I suppose is the critical one.
Here is a picture of my FBD:

Here I try to find the internal (in the critical cut) forces and moments by equilibrium:

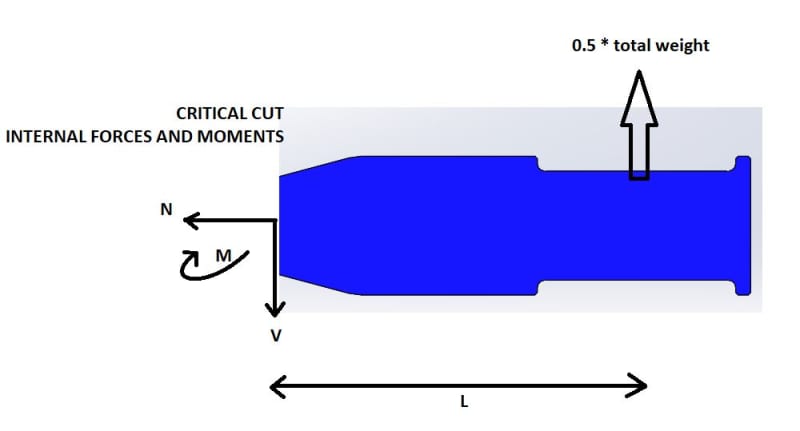
After finding V and M I find the shear stress and the normal stress using V/A and (M/I)*R correspondingly, and using von mizes relation sqrt(sigma^2 +3*tau^2) brings me to 80 Mpa.

The problem is that in the analysis I get around 25 Mpa.
I have to point out that I already checked a configuration in which there isn't a thread at all (as in the picture below), and the results didn't change.

So I am interested to know which one might be mistaken - the calculation or the analysis?
I just hope you can help me by checking the calculation process I made.

Thanks

 

[_eh-ngineer]

elinah34
There really aren't any relevant hand calculation formulas for a short beam. The closest you're going to get is something akin to stress on a gear tooth, and even this likely won't give you reasonable results for hand calcs.

FYI, this is all reviewable in Roark's Formulas for Stress and Strain section 8.10.

In this application you could consider only shear with a significant stress concentration at the shoulder.

 

[elinah34]

Thanks, I will take a look at it.